원환면 (torus)

개요

• genus 가 1인 컴팩트 유향곡면
• 복소함수론에서는 타원함수 를 위상적으로 원환면인 리만곡면에서 정의된 함수로 이해한다

매개화

• 매개화
• \(X(u,v)=\{\cos (u) (a+b \cos (v)),\sin (u) (a+b \cos (v)),b \sin (v)\}\)
  \(0<u<2\pi\), \(0<v<2\pi\)
  
• \(X_u=\{\sin (u) (-(a+b \cos (v))),\cos (u) (a+b \cos (v)),0\}\)
  \(X_v=\{-b \cos (u) \sin (v),-b \sin (u) \sin (v),b \cos (v)\}\)
  
• \(N=\{b \cos (u) \cos (v) (a+b \cos (v)),b \sin (u) \cos (v) (a+b \cos (v)),b \sin (v) (a+b \cos (v))\}\)
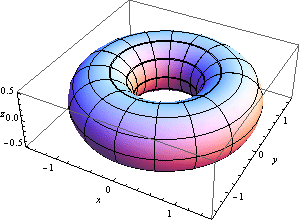
• 왼쪽 그림의 붉은 색 작은 원을 y-축에 대하여 회전하여, 오른쪽 원환면을 얻는다
  ->

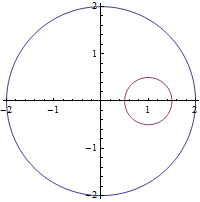제1기본형식

• \(E=(a+b \cos (v))^2\)
• \(F=0\)
• \(G=b^2\)

크리스토펠 기호

• 크리스토펠 기호 항목 참조
  \(\Gamma^1_{11}=0\)
  \(\Gamma^1_{12}=-\frac{b \sin (v)}{a+b \cos (v)}\)
  \(\Gamma^1_{21}=-\frac{b \sin (v)}{a+b \cos (v)}\)
  \(\Gamma^1_{22}=0\)
  \(\Gamma^2_{11}=\frac{\sin (v) (a+b \cos (v))}{b}\)
  \(\Gamma^2_{12}=0\)
  \(\Gamma^2_{21}=0\)
  \(\Gamma^2_{22}=0\)
  

측지선

• 측지선 이 만족시키는 미분방정식
  \(\frac{d^2\alpha_k }{dt^2} + \Gamma^{k}_{~i j }\frac{d\alpha_i }{dt}\frac{d\alpha_j }{dt} = 0\)
  
• 풀어쓰면,
  \(\frac{d^2 u}{dt^2} -\frac{2b \sin (v)}{a+b \cos (v)}\frac{du }{dt}\frac{dv }{dt} = 0\)
  \(\frac{d^2 v}{dt^2} + \frac{\sin (v) (a+b \cos (v))}{b}\frac{du }{dt}\frac{du }{dt} = 0\)
  

가우스곡률

• 가우스곡률 항목 참조
  \(K = -\frac{1}{2\sqrt{EG}}\left(\frac{\partial}{\partial u}\frac{G_u}{\sqrt{EG}} + \frac{\partial}{\partial v}\frac{E_v}{\sqrt{EG}}\right)=\frac{\cos (v)}{a b+b^2 \cos (v)}\)
  

역사

• http://www.google.com/search?hl=en&tbs=tl:1&q=
• 수학사연표

메모

• Math Overflow http://mathoverflow.net/search?q=

관련된 항목들

• 타원함수

매스매티카 파일 및 계산 리소스

• https://docs.google.com/leaf?id=0B8XXo8Tve1cxMDVmNGMxNzYtMjM5NC00ZWIwLWEwMzYtMGIwOWEwNTUwZDg0&sort=name&layout=list&num=50
• http://www.wolframalpha.com/input/?i=
• http://functions.wolfram.com/
• NIST Digital Library of Mathematical Functions
• Abramowitz and Stegun Handbook of mathematical functions
• The On-Line Encyclopedia of Integer Sequences
• Numbers, constants and computation
• 매스매티카 파일 목록

사전 형태의 자료

• http://ko.wikipedia.org/wiki/
• http://en.wikipedia.org/wiki/
• The Online Encyclopaedia of Mathematics
• NIST Digital Library of Mathematical Functions
• The World of Mathematical Equations

관련논문

• http://www.jstor.org/action/doBasicSearch?Query=
• http://www.ams.org/mathscinet
• http://dx.doi.org/