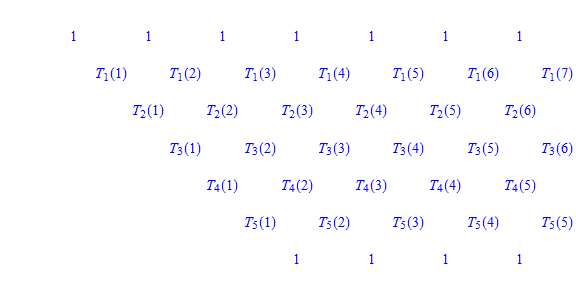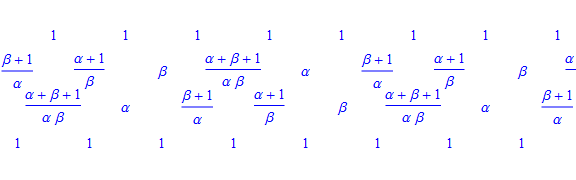"콕세터 프리즈"의 두 판 사이의 차이

수학노트
둘러보기로 가기 검색하러 가기
(새 문서: ==관련논문== * Conway, J. H., and H. S. M. Coxeter. 1973. “Triangulated Polygons and Frieze Patterns.” The Mathematical Gazette 57 (400) (June 1): 87–94. doi:10.2307/3615344...)
 
1번째 줄: 1번째 줄:
 +
==개요==
 +
* 자연수 $r$, $I=\{1\,\cdots, r\}$
 +
* 변수 $\left(Q^{(a)}_{m}\right)$, $m\in \mathbb{Z}_{\geq 0}$, $a\in I$에 대하여, 다음과 같은 점화식을 생각하자
 +
\begin{equation}
 +
\label{cf}
 +
\left\{
 +
\begin{array}{lll}
 +
Q^{(0)}_{m} =Q^{(r+1)}_{m} =1 & m\in \mathbb{Z}_{\geq 0} \\
 +
Q^{(a)}_{m}Q^{(a)}_{m+1}=1+Q^{(a-1)}_{m+1}Q^{(a+1)}_{m} & a\in I, m\in \mathbb{Z}_{\geq 0}
 +
\end{array}
 +
\right.
 +
\end{equation}
 +
* 아래의 그림은 $r=5$인 경우에 해당하며, $\left(Q^{(a)}_{1}\right)_{a\in I}$가 결정되면, 나머지 $Q^{(a)}_{m}$는 점화식 \ref{cf}로부터 얻어진다
 +
[[파일:콕세터 프리즈1.png]]
 +
 +
 +
* 점화식 \ref{cf}은 다음과 같은 배열이 $ad-bc=1$를 만족시키는 것으로 이해할 수 있다
 +
[[파일:콕세터 프리즈5.png]]
 +
==예==
 +
[[파일:콕세터 프리즈2.png]]
 +
 +
 +
[[파일:콕세터 프리즈3.png]]
 +
 +
 +
[[파일:콕세터 프리즈4.png]]
 +
 +
 
==관련논문==
 
==관련논문==
 
* Conway, J. H., and H. S. M. Coxeter. 1973. “Triangulated Polygons and Frieze Patterns.” The Mathematical Gazette 57 (400) (June 1): 87–94. doi:10.2307/3615344.
 
* Conway, J. H., and H. S. M. Coxeter. 1973. “Triangulated Polygons and Frieze Patterns.” The Mathematical Gazette 57 (400) (June 1): 87–94. doi:10.2307/3615344.
 
* Conway, J. H., and H. S. M. Coxeter. 1973. “Triangulated Polygons and Frieze Patterns (Continued).” The Mathematical Gazette 57 (401) (October 1): 175–183. doi:10.2307/3615561.
 
* Conway, J. H., and H. S. M. Coxeter. 1973. “Triangulated Polygons and Frieze Patterns (Continued).” The Mathematical Gazette 57 (401) (October 1): 175–183. doi:10.2307/3615561.
 
* Coxeter, H. S. M. 1971. “Frieze Patterns.” Polska Akademia Nauk. Instytut Matematyczny. Acta Arithmetica 18: 297–310. https://eudml.org/doc/204992
 
* Coxeter, H. S. M. 1971. “Frieze Patterns.” Polska Akademia Nauk. Instytut Matematyczny. Acta Arithmetica 18: 297–310. https://eudml.org/doc/204992

2013년 10월 25일 (금) 07:04 판

개요

  • 자연수 $r$, $I=\{1\,\cdots, r\}$
  • 변수 $\left(Q^{(a)}_{m}\right)$, $m\in \mathbb{Z}_{\geq 0}$, $a\in I$에 대하여, 다음과 같은 점화식을 생각하자

\begin{equation} \label{cf} \left\{ \begin{array}{lll} Q^{(0)}_{m} =Q^{(r+1)}_{m} =1 & m\in \mathbb{Z}_{\geq 0} \\ Q^{(a)}_{m}Q^{(a)}_{m+1}=1+Q^{(a-1)}_{m+1}Q^{(a+1)}_{m} & a\in I, m\in \mathbb{Z}_{\geq 0} \end{array} \right. \end{equation}

  • 아래의 그림은 $r=5$인 경우에 해당하며, $\left(Q^{(a)}_{1}\right)_{a\in I}$가 결정되면, 나머지 $Q^{(a)}_{m}$는 점화식 \ref{cf}로부터 얻어진다

콕세터 프리즈1.png


  • 점화식 \ref{cf}은 다음과 같은 배열이 $ad-bc=1$를 만족시키는 것으로 이해할 수 있다

콕세터 프리즈5.png

예

콕세터 프리즈2.png


콕세터 프리즈3.png


콕세터 프리즈4.png


관련논문

  • Conway, J. H., and H. S. M. Coxeter. 1973. “Triangulated Polygons and Frieze Patterns.” The Mathematical Gazette 57 (400) (June 1): 87–94. doi:10.2307/3615344.
  • Conway, J. H., and H. S. M. Coxeter. 1973. “Triangulated Polygons and Frieze Patterns (Continued).” The Mathematical Gazette 57 (401) (October 1): 175–183. doi:10.2307/3615561.
  • Coxeter, H. S. M. 1971. “Frieze Patterns.” Polska Akademia Nauk. Instytut Matematyczny. Acta Arithmetica 18: 297–310. https://eudml.org/doc/204992
원본 주소 "https://wiki.mathnt.net/index.php?title=콕세터_프리즈&oldid=28463"