"Kissing number and sphere packings"의 두 판 사이의 차이
둘러보기로 가기
검색하러 가기
잔글 (→저차원에서의 결과) |
Pythagoras0 (토론 | 기여) |
||
| (사용자 2명의 중간 판 7개는 보이지 않습니다) | |||
| 1번째 줄: | 1번째 줄: | ||
==개요== | ==개요== | ||
| − | + | ===Kissing number=== | |
| − | + | * 각 차원에서 주어진 구의 주변에 같은 크기의 구를 최대 몇 개까지 접하도록 배치할수 있는가의 문제 (주변의 구들은 서로 접할 수는 있으나 겹치는 것은 허용되지 않음) | |
| − | + | * 1차원에서는 2, 2차원에서는 6, 3차원에서는 12, 4차원에서는 24 | |
[[파일:1964116-2d.gif]] | [[파일:1964116-2d.gif]] | ||
| − | + | * 8차원에서는 240, 24차원에서는 196560 임이 알려져 있음. | |
| − | + | ** 이는 8차원의 [[E8]], 24차원의 리치(Leech)격자에 의해 얻어짐. | |
| − | + | * 나머지 차원은 아직 미해결. | |
| − | * | + | * "kissing"은 당구용어 ('''[ConwaySloane]''') |
| − | |||
| − | |||
| + | ===Sphere packings=== | ||
| + | * n차원 공간을 가장 효율적으로 채우는 구의 배치는 무엇인가의 문제 | ||
| + | * 일반적인 경우는 매우 어렵고, 좀더 접근이 가능한 경우인 격자 모양의 배치가 수학적으로 중요한 문제. | ||
==저차원에서의 kissing number에 관한 결과== | ==저차원에서의 kissing number에 관한 결과== | ||
| 97번째 줄: | 98번째 줄: | ||
==리뷰, 에세이, 강의노트== | ==리뷰, 에세이, 강의노트== | ||
| − | + | * Bezdek, Karoly, and Muhammad A. Khan. “Contact Numbers for Sphere Packings.” arXiv:1601.00145 [math], January 2, 2016. http://arxiv.org/abs/1601.00145. | |
| + | * Boyvalenkov, Peter, Stefan Dodunekov, and Oleg R. Musin. “A Survey on the Kissing Numbers.” arXiv:1507.03631 [math], July 13, 2015. http://arxiv.org/abs/1507.03631. | ||
* [http://www.ams.org/notices/200010/fea-elkies-1.pdf Lattices, Linear Codes and Invariants, Part I.] | * [http://www.ams.org/notices/200010/fea-elkies-1.pdf Lattices, Linear Codes and Invariants, Part I.] | ||
** Noam D. Elkies., 1238. NOTICES OF THE AMS. VOLUME. 47, NUMBER. 10. | ** Noam D. Elkies., 1238. NOTICES OF THE AMS. VOLUME. 47, NUMBER. 10. | ||
* [http://www.ams.org/notices/200011/fea-elkies-2.pdf Lattices, Linear Codes and Invariants,. Part II ] | * [http://www.ams.org/notices/200011/fea-elkies-2.pdf Lattices, Linear Codes and Invariants,. Part II ] | ||
** Noam D. Elkies., 1382. NOTICES OF THE AMS. VOLUME. 47, NUMBER. 11. | ** Noam D. Elkies., 1382. NOTICES OF THE AMS. VOLUME. 47, NUMBER. 11. | ||
| − | |||
| − | |||
| − | |||
==관련논문== | ==관련논문== | ||
| − | + | * Reid, Samuel. “On Contact Numbers of Finite Lattice Sphere Packings and the Maximal Coordination of Monatomic Crystals.” arXiv:1602.04246 [math], February 5, 2016. http://arxiv.org/abs/1602.04246. | |
* [http://arxiv.org/abs/0902.1105 High accuracy semidefinite programming bounds for kissing numbers] | * [http://arxiv.org/abs/0902.1105 High accuracy semidefinite programming bounds for kissing numbers] | ||
** Hans D. Mittelmann, Frank Vallentin, 2009 | ** Hans D. Mittelmann, Frank Vallentin, 2009 | ||
| 118번째 줄: | 117번째 줄: | ||
* [http://www.ams.org/notices/200408/fea-pfender.pdf Kissing numbers, sphere packings and some unexpected proofs] | * [http://www.ams.org/notices/200408/fea-pfender.pdf Kissing numbers, sphere packings and some unexpected proofs] | ||
** F. Pfender, G.M. Ziegler, Notices Amer. Math. Soc. 51 (8) (2004) 873-883. | ** F. Pfender, G.M. Ziegler, Notices Amer. Math. Soc. 51 (8) (2004) 873-883. | ||
| − | |||
| − | |||
==관련도서== | ==관련도서== | ||
| 127번째 줄: | 124번째 줄: | ||
** 이 분야의 가장 표준적인 도서 | ** 이 분야의 가장 표준적인 도서 | ||
* 케플러의 추측 | * 케플러의 추측 | ||
| + | |||
| + | ==메타데이터== | ||
| + | ===위키데이터=== | ||
| + | * ID : [https://www.wikidata.org/wiki/Q900117 Q900117] | ||
| + | ===Spacy 패턴 목록=== | ||
| + | * [{'LOWER': 'sphere'}, {'LEMMA': 'pack'}] | ||
2021년 2월 17일 (수) 03:48 기준 최신판
개요
Kissing number
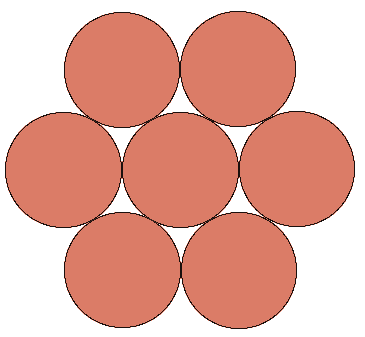
- 각 차원에서 주어진 구의 주변에 같은 크기의 구를 최대 몇 개까지 접하도록 배치할수 있는가의 문제 (주변의 구들은 서로 접할 수는 있으나 겹치는 것은 허용되지 않음)
- 1차원에서는 2, 2차원에서는 6, 3차원에서는 12, 4차원에서는 24
- 8차원에서는 240, 24차원에서는 196560 임이 알려져 있음.
- 이는 8차원의 E8, 24차원의 리치(Leech)격자에 의해 얻어짐.
- 나머지 차원은 아직 미해결.
- "kissing"은 당구용어 ([ConwaySloane])
Sphere packings
- n차원 공간을 가장 효율적으로 채우는 구의 배치는 무엇인가의 문제
- 일반적인 경우는 매우 어렵고, 좀더 접근이 가능한 경우인 격자 모양의 배치가 수학적으로 중요한 문제.
저차원에서의 kissing number에 관한 결과
1차원
- kissing number = 2
2차원
- kissing number = 6
3차원
- kissing number = 12
- 1694년 Isaac Newton 과 David Gregory가 이에 대해 토론함 ([ConwaySloane]).
- 1953년 Schütte와 van der Waerden에 의해 처음으로 제대로 증명됨 ([ConwaySloane])
- [Musin05]
4차원
- kissing number = 24
- 2003년 Oleg R. Musin에 의해 증명
- [Musin05], [Musin08]
고차원
- 5차원 이상에서는 8,24 차원을 제외하고 미해결
- 8차원에서는 240, 24차원에서는 196560 임이 알려져 있음.
- 이는 8차원의 E8, 24차원의 리치 격자(Leech lattice)에 의해 얻어짐.
메모
역사
- 1694, Newton and Gregory discussed if the solution in dimension 3
- http://www.google.com/search?hl=en&tbs=tl:1&q=kissing+number
- 수학사 연표
관련된 학부 과목과 미리 알고 있으면 좋은 것들
관련된 항목들
수학용어번역
- kissing - 대한수학회 수학용어집
사전 형태의 자료
리뷰, 에세이, 강의노트
- Bezdek, Karoly, and Muhammad A. Khan. “Contact Numbers for Sphere Packings.” arXiv:1601.00145 [math], January 2, 2016. http://arxiv.org/abs/1601.00145.
- Boyvalenkov, Peter, Stefan Dodunekov, and Oleg R. Musin. “A Survey on the Kissing Numbers.” arXiv:1507.03631 [math], July 13, 2015. http://arxiv.org/abs/1507.03631.
- Lattices, Linear Codes and Invariants, Part I.
- Noam D. Elkies., 1238. NOTICES OF THE AMS. VOLUME. 47, NUMBER. 10.
- Lattices, Linear Codes and Invariants,. Part II
- Noam D. Elkies., 1382. NOTICES OF THE AMS. VOLUME. 47, NUMBER. 11.
관련논문
- Reid, Samuel. “On Contact Numbers of Finite Lattice Sphere Packings and the Maximal Coordination of Monatomic Crystals.” arXiv:1602.04246 [math], February 5, 2016. http://arxiv.org/abs/1602.04246.
- High accuracy semidefinite programming bounds for kissing numbers
- Hans D. Mittelmann, Frank Vallentin, 2009
- [Musin08]The kissing number in four dimensions
- Oleg R. Musin, Annals of Mathematics, 168 (2008), No. 1, 1-32
- New upper bounds for kissing numbers from semidefinite programming
- Christine Bachoc, Frank Vallentin, 2007
- [Musin05]An extension of Delsarte's method. The kissing problem in three and four dimensions
- Oleg R. Musin, The Proceedings of COE Workshop on Sphere Packings (Nov. 1st - Nov. 5th, 2004), Kyushu University, Japan, 2005, 1-25
- Kissing numbers, sphere packings and some unexpected proofs
- F. Pfender, G.M. Ziegler, Notices Amer. Math. Soc. 51 (8) (2004) 873-883.
관련도서
- [ConwaySloane] Sphere Packings, Lattices and Groups (Grundlehren der mathematischen Wissenschaften)
- John Horton Conway, Neil J. A. Sloane
- 이 분야의 가장 표준적인 도서
- 케플러의 추측
메타데이터
위키데이터
- ID : Q900117
Spacy 패턴 목록
- [{'LOWER': 'sphere'}, {'LEMMA': 'pack'}]