"등시강하곡선 문제 (Tautochrone problem)"의 두 판 사이의 차이

수학노트
둘러보기로 가기 검색하러 가기
(피타고라스님이 이 페이지를 개설하였습니다.)
 
 
(사용자 2명의 중간 판 53개는 보이지 않습니다)
1번째 줄: 1번째 줄:
 +
==개요==
  
 +
* 중력을 받고 있는 물체가 출발점에 관계없이 주어진 목적지에 똑같은 시간에 도달하기 위해서 따라야 하는 곡선
 +
* [[사이클로이드]]에 의하여 만족됨
 +
* 1659년 호이겐스에 의해 해결
 +
* 진자 시계를 만드는데 활용되었다 http://hom.wikidot.com/the-cycloid
 +
 +
 +
 +
 +
 +
==등시성의 증명==
 +
 +
[[파일:4402517-Tautochrone_curve(1).gif]]
 +
 +
(정리) 사이클로이드를 따라 움직이는 추의 주기는 시작점의 위치에 관계없이 다음으로 주어진다.
 +
:<math>T =2\pi\sqrt{\frac{r}{g}}</math>
 +
 +
(이 때, 사이클로이드의 방정식은 <math>x = r(\theta - \sin \theta)</math>, <math>y = -r(1 - \cos \theta)</math>로 주어졌다고 하자.)
 +
 +
(증명)
 +
 +
출발점의 y좌표를 <math>y=y_0</math>라 두고, 그 때 곡선의 파라메터를 <math>\theta=\theta_0</math>라 하자.
 +
 +
움직이는 추의 속도는 <math>v=\sqrt{2g(y_0-y)}= \sqrt{2rg(\cos\theta_0-\cos\theta)}</math> 로 주어진다. 따라서 주기를 다음과 같이 쓸 수 있다
 +
:<math>T =\int \frac{ds}{v}=2\int_{\theta_0}^{\pi} \frac{\sqrt{2r^2(1-\cos\theta)}}{\sqrt{2rg(\cos\theta_0-\cos\theta)}}\,d\theta=2\sqrt{\frac{r}{g}}\int_{\theta_0}^{\pi} \frac{\sqrt{1-\cos\theta}}{\sqrt{\cos\theta_0-\cos\theta}}\,d\theta \label{vel}</math>
 +
 +
반각공식을 이용하여, \ref{vel}의 우변을 다음과 같이 쓸 수 있다
 +
 +
:<math>2\sqrt{\frac{r}{g}}\int_{\theta_0}^{\pi}\frac{\sin(\frac{1}{2}\theta)}{\sqrt{\cos^2(\frac{1}{2}\theta_0)-\cos^2(\frac{1}{2}\theta)}}d\theta </math>
 +
여기서
 +
:<math>u=\frac{\cos \frac{1}{2}\theta}{\cos \frac{1}{2}\theta_0}</math>로 치환하면,
 +
:<math>du=\frac{-\sin \frac{1}{2}\theta}{2\cos \frac{1}{2}\theta_0}\,d\theta</math> 를 얻는다.
 +
 +
따라서
 +
:<math>T =4\sqrt{\frac{r}{g}}\int_{0}^{1} \frac{1}{\sqrt{1-u^2}}\,du=2\pi\sqrt{\frac{r}{g}}</math>■
 +
 +
 +
 +
 +
==관련동영상==
 +
* 1분 15초 부터
 +
* http://www.youtube.com/watch?v=FAYWccuLVvY#t=1m15s
 +
 +
 +
 +
==메모==
 +
* http://www.baropage.com/file_board/view.php?id=life02&page=1&sn1=&divpage=1&sn=off&ss=on&sc=on&select_arrange=hit&desc=desc&no=95
 +
 +
 +
 +
 +
==메모==
 +
 +
* [http://www.math.nmsu.edu/%7Ehistory/mm-3-2-huygens.pdf http://www.math.nmsu.edu/~history/mm-3-2-huygens.pdf]
 +
* [http://lilith.gotdns.org/%7Evictor/writings/0033huygens.pdf Christiaan Huygens and the Scientific Revolution]
 +
 +
 +
 +
 +
 +
==관련된 항목들==
 +
* [[사이클로이드]]
 +
* [[단진자의 주기와 타원적분]]
 +
 +
 +
==매스매티카 파일 및 계산 리소스==
 +
* https://docs.google.com/file/d/0B8XXo8Tve1cxUGZ6TlpTemFuazg/edit
 +
 +
 +
 +
==수학용어번역==
 +
 +
*  Tautochrone problem
 +
** 등시강하곡선 문제
 +
 +
 +
 +
 +
==사전 형태의 자료==
 +
* http://en.wikipedia.org/wiki/Tautochrone_problem
 +
* http://mathworld.wolfram.com/TautochroneProblem.html
 +
 +
 +
 +
 +
==관련논문==
 +
 +
* [http://www.jstor.org/stable/2695647 The Cycloidal Pendulum]
 +
** Jeff Brooks and Satha Push, The American Mathematical Monthly Vol. 109, No. 5 (May, 2002), pp. 463-465
 +
 +
 +
[[분류:곡선]]
 +
 +
==메타데이터==
 +
===위키데이터===
 +
* ID :  [https://www.wikidata.org/wiki/Q3284444 Q3284444]
 +
===Spacy 패턴 목록===
 +
* [{'LOWER': 'tautochrone'}, {'LEMMA': 'curve'}]
 +
* [{'LOWER': 'isochrone'}, {'LEMMA': 'curve'}]

2021년 2월 17일 (수) 05:03 기준 최신판

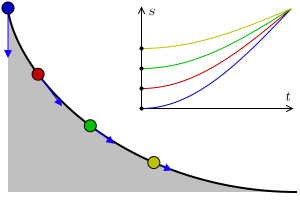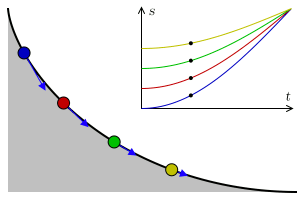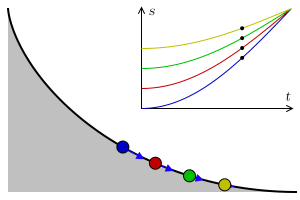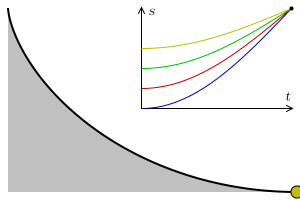
개요

  • 중력을 받고 있는 물체가 출발점에 관계없이 주어진 목적지에 똑같은 시간에 도달하기 위해서 따라야 하는 곡선
  • 사이클로이드에 의하여 만족됨
  • 1659년 호이겐스에 의해 해결
  • 진자 시계를 만드는데 활용되었다 http://hom.wikidot.com/the-cycloid



등시성의 증명

4402517-Tautochrone curve(1).gif

(정리) 사이클로이드를 따라 움직이는 추의 주기는 시작점의 위치에 관계없이 다음으로 주어진다. \[T =2\pi\sqrt{\frac{r}{g}}\]

(이 때, 사이클로이드의 방정식은 \(x = r(\theta - \sin \theta)\), \(y = -r(1 - \cos \theta)\)로 주어졌다고 하자.)

(증명)

출발점의 y좌표를 \(y=y_0\)라 두고, 그 때 곡선의 파라메터를 \(\theta=\theta_0\)라 하자.

움직이는 추의 속도는 \(v=\sqrt{2g(y_0-y)}= \sqrt{2rg(\cos\theta_0-\cos\theta)}\) 로 주어진다. 따라서 주기를 다음과 같이 쓸 수 있다 \[T =\int \frac{ds}{v}=2\int_{\theta_0}^{\pi} \frac{\sqrt{2r^2(1-\cos\theta)}}{\sqrt{2rg(\cos\theta_0-\cos\theta)}}\,d\theta=2\sqrt{\frac{r}{g}}\int_{\theta_0}^{\pi} \frac{\sqrt{1-\cos\theta}}{\sqrt{\cos\theta_0-\cos\theta}}\,d\theta \label{vel}\]

반각공식을 이용하여, \ref{vel}의 우변을 다음과 같이 쓸 수 있다

\[2\sqrt{\frac{r}{g}}\int_{\theta_0}^{\pi}\frac{\sin(\frac{1}{2}\theta)}{\sqrt{\cos^2(\frac{1}{2}\theta_0)-\cos^2(\frac{1}{2}\theta)}}d\theta \] 여기서 \[u=\frac{\cos \frac{1}{2}\theta}{\cos \frac{1}{2}\theta_0}\]로 치환하면, \[du=\frac{-\sin \frac{1}{2}\theta}{2\cos \frac{1}{2}\theta_0}\,d\theta\] 를 얻는다.

따라서 \[T =4\sqrt{\frac{r}{g}}\int_{0}^{1} \frac{1}{\sqrt{1-u^2}}\,du=2\pi\sqrt{\frac{r}{g}}\]■



관련동영상


메모



메모



관련된 항목들


매스매티카 파일 및 계산 리소스


수학용어번역

  • Tautochrone problem
    • 등시강하곡선 문제



사전 형태의 자료



관련논문

  • The Cycloidal Pendulum
    • Jeff Brooks and Satha Push, The American Mathematical Monthly Vol. 109, No. 5 (May, 2002), pp. 463-465

메타데이터

위키데이터

Spacy 패턴 목록

  • [{'LOWER': 'tautochrone'}, {'LEMMA': 'curve'}]
  • [{'LOWER': 'isochrone'}, {'LEMMA': 'curve'}]
원본 주소 "https://wiki.mathnt.net/index.php?title=등시강하곡선_문제_(Tautochrone_problem)&oldid=52235"