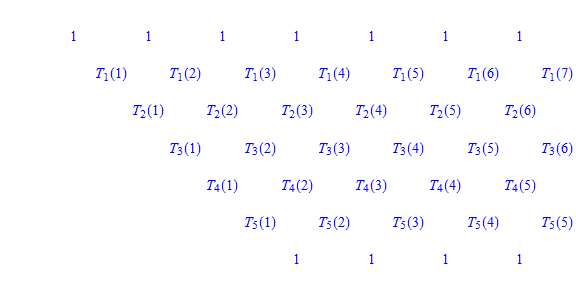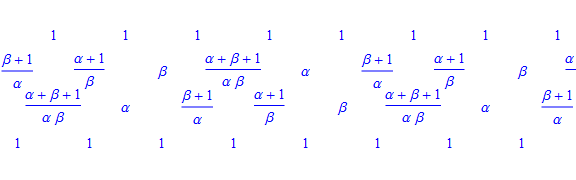콕세터 프리즈
개요
- $r$ : 자연수
- $I=\{1\,\cdots, r\}$
- 복소수 $T_{m}(u)$, $u\in \mathbb{Z}_{\geq 0}$, $m\in I$에 대하여, 다음과 같은 점화식을 생각하자
\begin{equation} \label{cf} \left\{ \begin{array}{lll} T_{0}(u) =T_{r+1}(u) =1 & u\in \mathbb{Z}_{\geq 0} \\ T_{m}(u)T_{m}(u+1)=1+T_{m-1}(u+1)T_{m+1}(u) & m\in I, u\in \mathbb{Z}_{\geq 0} \end{array} \right. \end{equation}
- 아래의 그림은 $r=5$인 경우에 해당하며, $\left(T_{m}(1)\right)_{m\in I}$가 결정되면, 나머지 $T_{m}(u)$는 점화식 \ref{cf}로부터 얻어진다
- 점화식 \ref{cf}은 다음과 같은 배열이 $ad-bc=1$를 만족시키는 것으로 이해할 수 있다
예
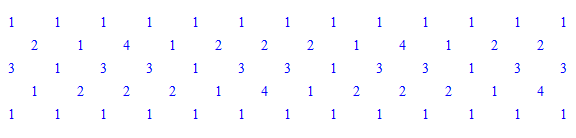
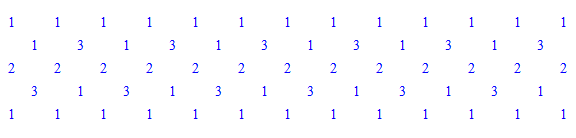
$r=2$
$r=3$
$r=4$
$r=6$
성질
주기성
- 각 $m\in I$에 대하여, $T_{m}(u+(r+3))=T_{m}(u)$이 성립한다
정수 수열
- 모든 $T_{m}(u)$, $u\in \mathbb{Z}_{\geq 0}$, $m\in I$가 정수가 될 필요충분조건은 다음과 같다
$$ T_{m}(1)|\left(T_{m-1}(1)+T_{m+1}(1)\right) $$
- (정리) 콕세터-콘웨이
모든 양의 정수로 이루어진 콕세터 프리즈는 정(r+3)-각형의 삼각화로부터 얻어진다 [H] 정리 4 참조
관련된 항목들
매스매티카 파일 및 계산 리소스
관련도서
- Coxeter, H. S. M. 1991. Regular Complex Polytopes. Cambridge [England]; New York: Cambridge University Press. http://www.amazon.com/Regular-Complex-Polytopes-H-Coxeter/dp/0521394902
- Chapter 5
리뷰, 에세이, 강의노트
- Morier-Genoud, Sophie. ‘Coxeter’s Frieze Patterns at the Crossroads of Algebra, Geometry and Combinatorics’. arXiv:1503.05049 Null, 17 March 2015. http://arxiv.org/abs/1503.05049.
관련논문
- Karin Baur, Klemens Fellner, Mark James Parsons, Manuela Tschabold, Growth behaviour of periodic tame friezes, http://arxiv.org/abs/1603.02127v1
- [H]Henry, Claire-Soizic. 2013. “Coxeter Friezes and Triangulations of Polygons.” American Mathematical Monthly 120 (6): 553–558. doi:10.4169/amer.math.monthly.120.06.553.
- Conway, J. H., and H. S. M. Coxeter. 1973. “Triangulated Polygons and Frieze Patterns.” The Mathematical Gazette 57 (400) (June 1): 87–94. doi:10.2307/3615344.
- Conway, J. H., and H. S. M. Coxeter. 1973. “Triangulated Polygons and Frieze Patterns (Continued).” The Mathematical Gazette 57 (401) (October 1): 175–183. doi:10.2307/3615561.
- Coxeter, H. S. M. 1971. “Frieze Patterns.” Polska Akademia Nauk. Instytut Matematyczny. Acta Arithmetica 18: 297–310. https://eudml.org/doc/204992