"쌍곡기하학"의 두 판 사이의 차이
둘러보기로 가기
검색하러 가기
Pythagoras0 (토론 | 기여) (→관련논문) |
|||
| (사용자 2명의 중간 판 14개는 보이지 않습니다) | |||
| 1번째 줄: | 1번째 줄: | ||
| − | + | ==개요== | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
* 일반적으로 비유클리드 기하학을 말할때 지칭하는 기하학 | * 일반적으로 비유클리드 기하학을 말할때 지칭하는 기하학 | ||
| − | * 한 직선<math>\ell</math>과 그 직선 위에 있지 않은 한 점<math>P</math> | + | * 쌍곡평면에서는 한 직선<math>\ell</math>과 그 직선 위에 있지 않은 한 점<math>P</math>가 주어져 있을때, <math>P</math>를 지나는 <math>\ell</math>과 평행한 직선이 무수히 많이 존재 |
* 곡률이 음인 공간에서의 기하학을 지칭하는 말 | * 곡률이 음인 공간에서의 기하학을 지칭하는 말 | ||
| − | * 곡률이 음인 공간은 3차원 상에서 각 점이 말안장처럼 보이게 | + | * 곡률이 음인 공간은 3차원 상에서 각 점이 말안장처럼 보이게 된다 |
| − | + | * 쌍곡면은 쌍곡기하학의 모델을 제공하므로 쌍곡기하학이라 부르게 되었다 | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | * | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | ==쌍곡기하학의 두가지 모델== | |
| + | ====푸앵카레 상반평면 모델==== | ||
| + | * <math>\mathbb{H}^2=\{z=x+iy\in\mathbb{C}|y>0\}</math>:<math>ds^2=\frac{dx^2+dy^2}{y^2}=\frac{dzd\overline{z}}{y^2}</math> | ||
| + | * [[푸앵카레 상반평면 모델]]에서 자세히 다룸 | ||
| − | |||
| − | + | ====푸앵카레 unit disk 모델==== | |
| + | * [[푸앵카레 unit disk 모델]] | ||
| + | :<math>U=\{z=x+iy:|z|=\sqrt{x^2+y^2} < 1 \}</math> | ||
| + | :<math>ds^2=\frac{dx^2+dy^2}{(1-(x^2+y^2))^2}=\frac{dz\,d\overline{z}}{(1-|z|^2)^2}</math> | ||
| − | + | ==쌍곡기하학의 테셀레이션== | |
| + | * (2,3,7)-삼각형을 이용한 테셀레이션 | ||
| + | * (2,3,7)이란 삼각형의 세 각이 각각 :<math>\frac{\pi}{7},\frac{\pi}{3},\frac{\pi}{2}</math> | ||
| + | 라는 것을 말한다. 이 세각의 크기를 모두 더하면,:<math>\frac{\pi}{7}+\frac{\pi}{3}+\frac{\pi}{2}=\frac{41\pi}{42}</math> | ||
| + | 가 되어, 180도보다 작게 됨을 알 수 있다. '''쌍곡기하학에서의 곡률은 음수이기 때문에 나타나는 현상이다.''' | ||
| + | [[파일:3065168-H2PlaneLines_med.jpg]] | ||
| + | * [[2차원 쌍곡기하학의 테셀레이션]] 항목 참조 | ||
| − | |||
| − | + | ==역사== | |
* 1824년 가우스의 쌍곡기하학에 대한 연구 | * 1824년 가우스의 쌍곡기하학에 대한 연구 | ||
| − | * 1829년 | + | * 1829년 로바체프스키 [[쌍곡기하학]]에 대한 출판 |
* 1832년 볼리아이 | * 1832년 볼리아이 | ||
* 1868년 벨트라미는 비유클리드 기하학이 음의 곡률을 갖는 곡면위의 기하학임을 보임 | * 1868년 벨트라미는 비유클리드 기하학이 음의 곡률을 갖는 곡면위의 기하학임을 보임 | ||
* '''[Milnor1982]''' | * '''[Milnor1982]''' | ||
| − | * [[ | + | * [[수학사 연표]] |
| − | |||
| − | |||
| − | + | ||
| − | |||
| − | + | ||
| − | + | ==관련된 항목들== | |
| − | |||
| − | |||
* [[미분기하학]] | * [[미분기하학]] | ||
| 126번째 줄: | 49번째 줄: | ||
* [[교차비(cross ratio)|사영기하학과 교차비]] | * [[교차비(cross ratio)|사영기하학과 교차비]] | ||
* [[fundamental domain의 면적에 대한 지겔의 정리]] | * [[fundamental domain의 면적에 대한 지겔의 정리]] | ||
| − | * [[모듈라 군, j-invariant and the singular moduli]][[모듈라 군, j-invariant and the singular moduli|]] | + | * [[모듈라 군, j-invariant and the singular moduli]][[모듈라 군, j-invariant and the singular moduli|모듈라 군]] |
** [[모듈라 군(modular group)]] | ** [[모듈라 군(modular group)]] | ||
* [[수학과 미술]] | * [[수학과 미술]] | ||
| − | + | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | ||
| − | + | ==사전 형태의 자료== | |
| − | |||
* http://ko.wikipedia.org/wiki/ | * http://ko.wikipedia.org/wiki/ | ||
| − | * | + | * http://en.wikipedia.org/wiki/Poincaré_half-plane_model |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | ||
| − | + | ==리뷰, 에세이, 강의노트== | |
| + | * Mahan Mj, What is hyperbolic geometry? Asia Pacific Mathematics Newsletter 2013, http://www.asiapacific-mathnews.com/03/0301/0001_0005.pdf | ||
| + | * James W. Cannon, William J. Floyd, Richard Kenyon, and Walter R. Parry, [http://www.msri.org/communications/books/Book31/files/cannon.pdf Hyperbolic Geometry], 1997, MSRI Publications, volume 31. | ||
| + | * Shenitzer, Abe. “How Hyperbolic Geometry Became Respectable.” The American Mathematical Monthly 101, no. 5 (May 1, 1994): 464–70. doi:10.2307/2974912. | ||
| + | * '''[Milnor1982]''', John W. Milnor [http://projecteuclid.org/euclid.bams/1183548588 Hyperbolic geometry: The first 150 years], Journal: Bull. Amer. Math. Soc. 6 (1982), 9-24. | ||
| − | + | ==관련도서== | |
| − | * [http://www.amazon.com/Gateway-Modern-Geometry-Poincare-Half-Plane/dp/0763753815/ref=sr_1_1?ie=UTF8&s=books&qid=1259658855&sr=1-1 Poincare Half-Plane (A Gateway to Modern Geometry)] | + | * [http://www.amazon.com/Gateway-Modern-Geometry-Poincare-Half-Plane/dp/0763753815/ref=sr_1_1?ie=UTF8&s=books&qid=1259658855&sr=1-1 Poincare Half-Plane (A Gateway to Modern Geometry)] |
** S. Stahl, Jones & Bartlett Publishers; 2 edition (November 25, 2007) | ** S. Stahl, Jones & Bartlett Publishers; 2 edition (November 25, 2007) | ||
| − | * [http://www.amazon.com/Sources-Hyperbolic-Geometry-History-Mathematics/dp/0821809229 Sources of Hyperbolic Geometry] | + | * [http://www.amazon.com/Sources-Hyperbolic-Geometry-History-Mathematics/dp/0821809229 Sources of Hyperbolic Geometry] |
** John Stillwell, American Mathemataical Society (December 1996) | ** John Stillwell, American Mathemataical Society (December 1996) | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | ||
| + | |||
| − | + | ==블로그== | |
| − | * 피타고라스의 창 | + | * 피타고라스의 창 |
** [http://bomber0.byus.net/index.php/2008/10/03/799 비유클리드 기하학 입문(1) : 에셔의 CIRCLE LIMIT 시리즈] | ** [http://bomber0.byus.net/index.php/2008/10/03/799 비유클리드 기하학 입문(1) : 에셔의 CIRCLE LIMIT 시리즈] | ||
** [http://bomber0.byus.net/index.php/2008/10/03/806 비유클리드 기하학 입문(2) : 휘어진 공간] | ** [http://bomber0.byus.net/index.php/2008/10/03/806 비유클리드 기하학 입문(2) : 휘어진 공간] | ||
** [http://bomber0.byus.net/index.php/2008/10/11/821 비유클리드 기하학 입문(3) : 콕세터가 들려주는 에셔] | ** [http://bomber0.byus.net/index.php/2008/10/11/821 비유클리드 기하학 입문(3) : 콕세터가 들려주는 에셔] | ||
** [http://bomber0.byus.net/index.php/2008/10/22/836 비유클리드 기하학 입문(4) : 콕세터가 설명하는 반전(inversion)] | ** [http://bomber0.byus.net/index.php/2008/10/22/836 비유클리드 기하학 입문(4) : 콕세터가 설명하는 반전(inversion)] | ||
| − | ** [http://bomber0.byus.net/index.php/2008/10/22/839 비유클리드 기하학 입문(5) : 반전에 반전 | + | ** [http://bomber0.byus.net/index.php/2008/10/22/839 비유클리드 기하학 입문(5) : 반전에 반전 \[Ellipsis] 반전만 구백번\[Ellipsis]] |
** [http://bomber0.byus.net/index.php/2008/11/14/872 비유클리드 기하학 입문(6) : 세가지의 2차원 기하학] | ** [http://bomber0.byus.net/index.php/2008/11/14/872 비유클리드 기하학 입문(6) : 세가지의 2차원 기하학] | ||
| − | + | [[분류:쌍곡기하학]] | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
2014년 12월 31일 (수) 08:52 판
개요
- 일반적으로 비유클리드 기하학을 말할때 지칭하는 기하학
- 쌍곡평면에서는 한 직선\(\ell\)과 그 직선 위에 있지 않은 한 점\(P\)가 주어져 있을때, \(P\)를 지나는 \(\ell\)과 평행한 직선이 무수히 많이 존재
- 곡률이 음인 공간에서의 기하학을 지칭하는 말
- 곡률이 음인 공간은 3차원 상에서 각 점이 말안장처럼 보이게 된다
- 쌍곡면은 쌍곡기하학의 모델을 제공하므로 쌍곡기하학이라 부르게 되었다
쌍곡기하학의 두가지 모델
푸앵카레 상반평면 모델
- \(\mathbb{H}^2=\{z=x+iy\in\mathbb{C}|y>0\}\)\[ds^2=\frac{dx^2+dy^2}{y^2}=\frac{dzd\overline{z}}{y^2}\]
- 푸앵카레 상반평면 모델에서 자세히 다룸
푸앵카레 unit disk 모델
\[U=\{z=x+iy:|z|=\sqrt{x^2+y^2} < 1 \}\] \[ds^2=\frac{dx^2+dy^2}{(1-(x^2+y^2))^2}=\frac{dz\,d\overline{z}}{(1-|z|^2)^2}\]
쌍곡기하학의 테셀레이션
- (2,3,7)-삼각형을 이용한 테셀레이션
- (2,3,7)이란 삼각형의 세 각이 각각 \[\frac{\pi}{7},\frac{\pi}{3},\frac{\pi}{2}\]
라는 것을 말한다. 이 세각의 크기를 모두 더하면,\[\frac{\pi}{7}+\frac{\pi}{3}+\frac{\pi}{2}=\frac{41\pi}{42}\]
가 되어, 180도보다 작게 됨을 알 수 있다. 쌍곡기하학에서의 곡률은 음수이기 때문에 나타나는 현상이다.
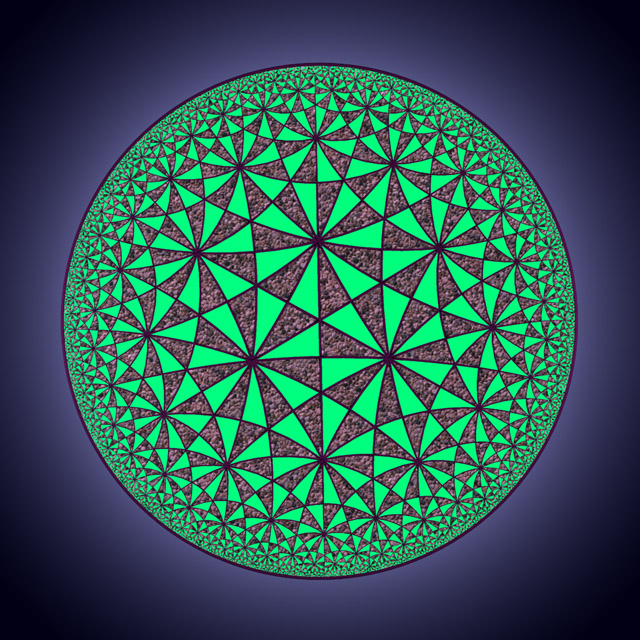
- 2차원 쌍곡기하학의 테셀레이션 항목 참조
역사
- 1824년 가우스의 쌍곡기하학에 대한 연구
- 1829년 로바체프스키 쌍곡기하학에 대한 출판
- 1832년 볼리아이
- 1868년 벨트라미는 비유클리드 기하학이 음의 곡률을 갖는 곡면위의 기하학임을 보임
- [Milnor1982]
- 수학사 연표
관련된 항목들
- 미분기하학
- 뫼비우스 변환군과 기하학
- 로바체프스키와 클라우센 함수
- 사영기하학과 교차비
- fundamental domain의 면적에 대한 지겔의 정리
- 모듈라 군, j-invariant and the singular moduli모듈라 군
- 수학과 미술
사전 형태의 자료
리뷰, 에세이, 강의노트
- Mahan Mj, What is hyperbolic geometry? Asia Pacific Mathematics Newsletter 2013, http://www.asiapacific-mathnews.com/03/0301/0001_0005.pdf
- James W. Cannon, William J. Floyd, Richard Kenyon, and Walter R. Parry, Hyperbolic Geometry, 1997, MSRI Publications, volume 31.
- Shenitzer, Abe. “How Hyperbolic Geometry Became Respectable.” The American Mathematical Monthly 101, no. 5 (May 1, 1994): 464–70. doi:10.2307/2974912.
- [Milnor1982], John W. Milnor Hyperbolic geometry: The first 150 years, Journal: Bull. Amer. Math. Soc. 6 (1982), 9-24.
관련도서
- Poincare Half-Plane (A Gateway to Modern Geometry)
- S. Stahl, Jones & Bartlett Publishers; 2 edition (November 25, 2007)
- Sources of Hyperbolic Geometry
- John Stillwell, American Mathemataical Society (December 1996)