"포드 원 (Ford Circles)"의 두 판 사이의 차이

수학노트
둘러보기로 가기 검색하러 가기
 
(사용자 2명의 중간 판 14개는 보이지 않습니다)
1번째 줄: 1번째 줄:
−
<h5 style="margin: 0px; line-height: 3.428em; color: rgb(34, 61, 103); font-family: 'malgun gothic',dotum,gulim,sans-serif; font-size: 1.166em; background-position: 0px 100%;">이 항목의 스프링노트 원문주소</h5>
+
==개요==
 +
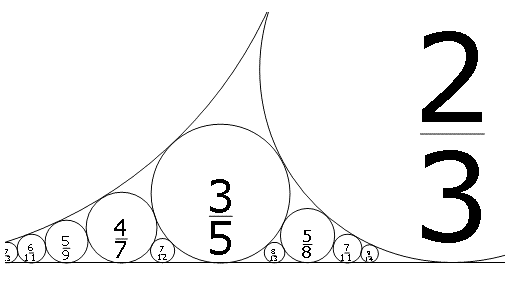
* <math>p,q</math>가 서로 소인 자연수일 때, 중심이 <math>(\frac{p}{q},\frac{1}{2q^2})</math> 이고, 반지름이 <math>\frac{1}{2q^2}</math>인 원을 포드 원이라 함
 +
* <math>x</math>-축에 접한다
  
−
* [[포드 원 (Ford Circles)]]
+
[[파일:포드 원 (Ford Circles)1.gif]]
  
−
 
 
  
−
 
+
==관찰==
 +
* 위 그림을 잘 보면서 관찰해 보자. (원 안에 적혀 있는 숫자는, 원 중심의 <math>x</math> 좌표이다.)
 +
* <math>p,q</math>가 서로소인 자연수이므로, 원 중심의 <math>x</math> 좌표들은 기약분수이다.
 +
* 서로 다른 두 포드 원은 만나지 않거나, 접한다.
 +
* 접하는 두 포드 원 사이에는 어떤 관계가 있을까?
 +
** <math>\frac35 , \frac23</math>
 +
** <math>\frac35 , \frac58</math>
 +
** <math>\frac58, \frac23</math>
 +
** <math>\frac58, \frac{7}{11}</math>
 +
* 서로 접하는 세 포드 원 사이에는 어떤 관계가 있을까
 +
** <math>\frac35, \frac58 , \frac23</math>
 +
** <math>\frac35, \frac{8}{13} , \frac58</math>
 +
** <math>\frac58, \frac{7}{11} , \frac23</math>
 +
** <math>\frac47, \frac{7}{12} , \frac35</math>
 +
* [[패리 수열(Farey series)]]
  
−
<h5>개요</h5>
+
==관찰의 증명==
  
−
 
+
;정리
 +
두 포드 원은 만나지 않거나, 접한다.
  
−
[[Media:|]]
+
;증명
−
 
 
−
* <math>p,q</math>가 서로 소인 자연수일 때, 중심이 <math>(\frac{p}{q},\frac{1}{2q^2})</math> 이고, 반지름이 <math>\frac{1}{2q^2}</math>인 원을 포드 원이라 함<br>
 
−
** <math>y=0</math>에 접함
 
−
 
 
−
 
 
−
 
 
−
[/pages/3210238/attachments/5216304 ford_circles.gif]
 
−
 
 
−
 
 
−
 
 
−
 
 
−
 
 
−
<h5>관찰</h5>
 
−
 
 
−
위 그림을 잘 보면서 관찰해 보자. (원 안에 적혀 있는 숫자는, 원 중심의 <math>x</math> 좌표이다.)
 
−
 
 
−
 
 
−
 
 
−
* <math>p,q</math>가 서로소인 자연수들이니까, 원 중심의 <math>x</math> 좌표들은 기약분수들이 되겠다.
 
−
* 서로 겹치는 두 Ford circle 은 없는 듯 하다.
 
−
*  접하는 두 포드 원 사이에는 어떤 관계가 있을까?<br>
 
−
** <math>\frac35 , \frac23</math>      <math>\frac35 , \frac58</math>      <math>\frac58, \frac23</math>      <math>\frac58, \frac{7}{11}</math>   ...
 
−
** <math>10-9 = 25-24 = 16 - 15 = 56 - 55 = \cdots = 1</math>
 
−
*  서로 접하는 세 포드 원 사이에는?<br>
 
−
** <math>\frac35, \frac58 , \frac23</math>      <math>\frac35, \frac{8}{13} , \frac58</math>      <math>\frac58, \frac{7}{11} , \frac23</math>      <math>\frac47, \frac{7}{12} , \frac35</math>
 
−
** 뭔가 발견했는가?
 
−
 
 
−
이제 [[패리 수열(Farey series)|Farey series]] 를 읽고 다시 돌아오자. (오른쪽 클릭 - 새 탭 열기/새 창 열기)
 
−
 
 
−
* 서로 접하는 세 원의 중심의 <math>x</math> 좌표를 보자. 저 세 수를 가지는 (가장 작은) Farey Series 를 찾을 수 있겠는가? 그 때, 그 세 수는 어떻게 배열되어 있는가?
 
−
 
 
−
 
 
−
 
 
−
 
 
−
 
 
−
<h5>관찰의 증명</h5>
 
−
 
 
−
# 서로 겹치는 두 포드 원은 없음.
 
−
 
 
−
Proof.
 
  
 
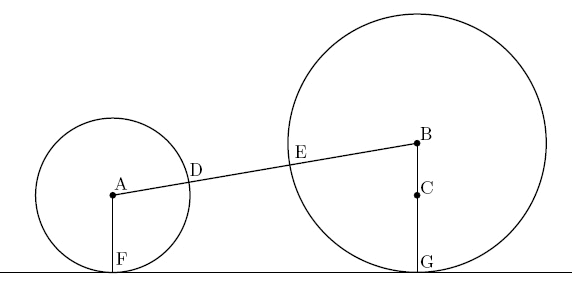
아래에 서로 다른 두 개의 포드 원을 그렸다. 원 A 는 중심의 <math>x</math> 좌표가 <math>p/q</math> 인 원이고, 원 B 는 중심의 <math>x</math> 좌표가 <math>P/Q</math> 인 원이다. (<math>p,q, P, Q</math> 는 자연수, <math>gcd(p,q) = gcd(P, Q) = 1</math>)
 
아래에 서로 다른 두 개의 포드 원을 그렸다. 원 A 는 중심의 <math>x</math> 좌표가 <math>p/q</math> 인 원이고, 원 B 는 중심의 <math>x</math> 좌표가 <math>P/Q</math> 인 원이다. (<math>p,q, P, Q</math> 는 자연수, <math>gcd(p,q) = gcd(P, Q) = 1</math>)
  
−
[/pages/3210238/attachments/2009497 fig1.jpg]
+
[[파일:포드 원 (Ford Circles)3.gif]]
−
 
 
−
 
 
  
 +
 
위 그림에서, 점 <math>A</math> 에서 선분 <math>\overline{BG}</math> 위에 내린 발을 <math>C</math> 라 하자. 그러면 삼각형 <math>\triangle ACB</math> 는 직각삼각형이 된다. 피타고라스의 정리를 적용하면,
 
위 그림에서, 점 <math>A</math> 에서 선분 <math>\overline{BG}</math> 위에 내린 발을 <math>C</math> 라 하자. 그러면 삼각형 <math>\triangle ACB</math> 는 직각삼각형이 된다. 피타고라스의 정리를 적용하면,
  
−
<math>\overline{AB}^2 = \overline{BC}^2 + \overline{CA}^2</math> 이다. 포드 원의 정의에서 <math>A(\frac{p}{q}, \frac{1}{2q^2}), B(\frac{P}{Q}, \frac{1}{2Q^2}), C(\frac{P}{Q},\frac{1}{2q^2} )</math> 이므로, 각 변의 길이를 구해서 정리하면 <math>\overline{AB}^2 = (\overline{AD} + \overline{EB})^2 + \frac{(Pq - pQ)^2 - 1}{Q^2 q^2}</math> 를 얻는다. (http://www.wolframalpha.com/input/?i=%28P%2FQ+-+p%2Fq%29%5E2+%2B+%281%2F%282+Q%5E2%29+-+1%2F%282+q%5E2%29%29%5E2+-+%281%2F%282+q%5E2%29+%2B+1%2F%282+Q%5E2%29%29%5E2+%2F%2F+FullSimplify )
+
<math>\overline{AB}^2 = \overline{BC}^2 + \overline{CA}^2</math> 이다. 포드 원의 정의에서 <math>A(\frac{p}{q}, \frac{1}{2q^2}), B(\frac{P}{Q}, \frac{1}{2Q^2}), C(\frac{P}{Q},\frac{1}{2q^2} )</math> 이므로, 다음이 성립한다
 +
:<math>
 +
\overline{AB}^2 = (\overline{AD} + \overline{EB})^2 + \frac{(Pq - pQ)^2 - 1}{Q^2 q^2}
 +
</math>
  
 
여기서,
 
여기서,
  
−
i.  <math>|Pq -pQ|> 1</math> 이면, <math>\overline{AB} > \overline{AD} + \overline{EB}</math> 이므로, 두 원은 서로 떨어져 있다.
+
i. <math>|Pq -pQ|> 1</math> 이면, <math>\overline{AB} > \overline{AD} + \overline{EB}</math> 이므로, 두 원은 서로 떨어져 있다.
  
−
ii.  <math>|Pq -pQ|= 1</math> 이면, <math>\overline{AB} = \overline{AD} + \overline{EB}</math> 이므로, 두 원은 접한다. (10-나 과정의 '두 원의 위치 관계' 참조)
+
ii. <math>|Pq -pQ|= 1</math> 이면, <math>\overline{AB} = \overline{AD} + \overline{EB}</math> 이므로, 두 원은 접한다.
  
 
iii. <math>|Pq -pQ| <1</math> 일 수는 없다.
 
iii. <math>|Pq -pQ| <1</math> 일 수는 없다.
73번째 줄: 51번째 줄:
 
왜냐하면, <math>p,q, P, Q</math> 는 자연수이므로 <math>Pq -pQ = 0</math> 이면, <math>p/q \ne P/Q</math> 에 모순이기 때문이다.
 
왜냐하면, <math>p,q, P, Q</math> 는 자연수이므로 <math>Pq -pQ = 0</math> 이면, <math>p/q \ne P/Q</math> 에 모순이기 때문이다.
  
−
위 세 가지 경우에서, 서로 겹쳐 있는 두 포드 원은 없음을 알 수 있다.
+
위 세 가지 경우에서, 서로 겹쳐 있는 두 포드 원은 없음을 알 수 있다. ■
  
−
 
 
  
−
2. 접하는 두 포드 원 사이의 관계
+
;정리
 +
<math>x</math> 좌표가 <math>p/q</math> 인 포드 원을 <math>C[p/q]</math> 라고 쓰자. 두 포드 원 <math>C[b/a]</math> 과 <math>C[d/c]</math>이 접하면, <math>|ad - bc| = 1</math> 이 성립한다.
  
−
<math>x</math> 좌표가 <math>p/q</math> 인 포드 원을 <math>C[p/q]</math> 라고 쓰자.
+
;증명
  
−
접하는 두 포드 원 <math>C[b/a]</math> 과 <math>C[d/c]</math> 가 있으면, <math>|ad - bc| = 1</math> 이다.
+
관찰 1 의 증명 중 ii) 로부터 알 수 있다. ■
  
−
Proof.
+
3. Farey Series 와의 관계
  
−
관찰 1 의 증명 중 ii) 로부터 알 수 있다.
 
  
−
3. Farey Series 와의 관계
+
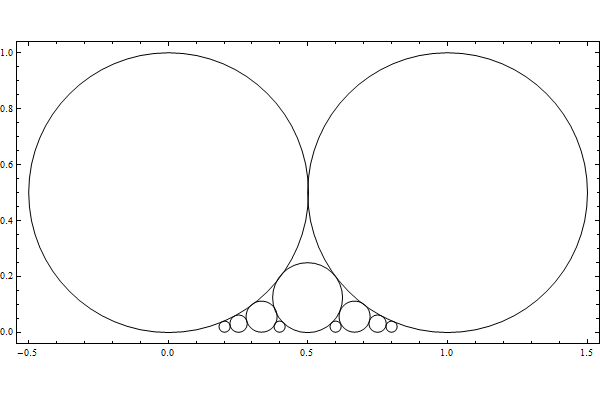
==메모==
 +
[[파일:포드 원 (Ford Circles)2.gif]]
  
−
 
 
  
−
 
 
  
−
<h5>관련된 항목들</h5>
+
==관련된 항목들==
  
−
* [[패리 수열(Farey series)|Farey series]]
+
* [[패리 수열(Farey series)]]
−
* [[모듈라 군, j-invariant and the singular moduli|The modular group, j-invariant and the singular moduli]]<br>
+
* [[모듈라 군, j-invariant and the singular moduli]]
−
** [[모듈라 군(modular group)|modular group]]
+
* [[모듈라 군(modular group)]]
 
* [[연분수와 유리수 근사]]
 
* [[연분수와 유리수 근사]]
  
−
 
+
  
−
 
+
==매스매티카 파일 및 계산 리소스==
  
−
<h5>매스매티카 파일 및 계산 리소스</h5>
+
* https://drive.google.com/file/d/0B8XXo8Tve1cxNmU5NDhlZGMtYWU5MS00YThiLWFiZjEtNjk4N2FmZWI5ZmQy/view
 +
* http://mathematica.stackexchange.com/questions/100628/wiggly-and-imprecise-animated-gif-output
  
−
* [[3210238/attachments/5216306|포드_원_(Ford_Circles).nb]]
+
==사전형태의 자료==
−
* http://www.wolframalpha.com/input/?i=
 
−
* http://functions.wolfram.com/
 
−
* [http://dlmf.nist.gov/ NIST Digital Library of Mathematical Functions]
 
−
* [http://www.research.att.com/%7Enjas/sequences/index.html The On-Line Encyclopedia of Integer Sequences]
 
−
* [http://numbers.computation.free.fr/Constants/constants.html Numbers, constants and computation]
 
−
 
 
−
* [[매스매티카 파일 목록]]
 
−
 
 
−
 
 
−
 
 
−
 
 
−
 
 
−
 
 
−
 
 
−
<h5>사전형태의 자료</h5>
 
  
 
* http://en.wikipedia.org/wiki/Ford_circles
 
* http://en.wikipedia.org/wiki/Ford_circles
  
−
 
+
−
 
+
==리뷰, 에세이, 강의노트==
−
 
 
−
 
 
−
<h5>리뷰논문, 에세이, 강의노트</h5>
 
  
−
* [[3210238/attachments/1980825|Ford_Circle.pdf]]<br>
+
* 애기똥풀, [[파일:3210238-Ford Circle.pdf]]
−
** 애기똥풀
+
* 이광연, [http://navercast.naver.com/science/math/1049 바보셈에서 페리수열], 네이버 오늘의 과학, 2009년 9월 8일
−
* [http://navercast.naver.com/science/math/1049 바보셈에서 페리수열]<br>
 
−
** 네이버 오늘의 과학, 2009년 9월 8일, 이광연
 
  
−
 
+
  
−
 
 
  
−
<h5>관련논문</h5>
+
==관련논문==
 +
* Athreya, Jayadev, Sneha Chaubey, Amita Malik, and Alexandru Zaharescu. “Geometry of Farey-Ford Polygons.” arXiv:1410.4908 [math], October 18, 2014. http://arxiv.org/abs/1410.4908.
 +
* [http://www.jstor.org/stable/2302799 Fractions] L. R. Ford, <cite>The American Mathematical Monthly</cite>, Vol. 45, No. 9 (Nov., 1938), pp. 586-601
  
−
* Ford193
+
==메타데이터==
−
* [http://www.jstor.org/stable/2302799 Fractions] L. R. Ford, <cite>The American Mathematical Monthly</cite>, Vol. 45, No. 9 (Nov., 1938), pp. 586-601
+
===위키데이터===
 +
* ID :  [https://www.wikidata.org/wiki/Q1436714 Q1436714]
 +
===Spacy 패턴 목록===
 +
* [{'LOWER': 'ford'}, {'LEMMA': 'circle'}]

2021년 2월 17일 (수) 06:06 기준 최신판

개요

  • \(p,q\)가 서로 소인 자연수일 때, 중심이 \((\frac{p}{q},\frac{1}{2q^2})\) 이고, 반지름이 \(\frac{1}{2q^2}\)인 원을 포드 원이라 함
  • \(x\)-축에 접한다

포드 원 (Ford Circles)1.gif


관찰

  • 위 그림을 잘 보면서 관찰해 보자. (원 안에 적혀 있는 숫자는, 원 중심의 \(x\) 좌표이다.)
  • \(p,q\)가 서로소인 자연수이므로, 원 중심의 \(x\) 좌표들은 기약분수이다.
  • 서로 다른 두 포드 원은 만나지 않거나, 접한다.
  • 접하는 두 포드 원 사이에는 어떤 관계가 있을까?
    • \(\frac35 , \frac23\)
    • \(\frac35 , \frac58\)
    • \(\frac58, \frac23\)
    • \(\frac58, \frac{7}{11}\)
  • 서로 접하는 세 포드 원 사이에는 어떤 관계가 있을까
    • \(\frac35, \frac58 , \frac23\)
    • \(\frac35, \frac{8}{13} , \frac58\)
    • \(\frac58, \frac{7}{11} , \frac23\)
    • \(\frac47, \frac{7}{12} , \frac35\)
  • 패리 수열(Farey series)

관찰의 증명

정리

두 포드 원은 만나지 않거나, 접한다.

증명

아래에 서로 다른 두 개의 포드 원을 그렸다. 원 A 는 중심의 \(x\) 좌표가 \(p/q\) 인 원이고, 원 B 는 중심의 \(x\) 좌표가 \(P/Q\) 인 원이다. (\(p,q, P, Q\) 는 자연수, \(gcd(p,q) = gcd(P, Q) = 1\))

포드 원 (Ford Circles)3.gif


위 그림에서, 점 \(A\) 에서 선분 \(\overline{BG}\) 위에 내린 발을 \(C\) 라 하자. 그러면 삼각형 \(\triangle ACB\) 는 직각삼각형이 된다. 피타고라스의 정리를 적용하면,

\(\overline{AB}^2 = \overline{BC}^2 + \overline{CA}^2\) 이다. 포드 원의 정의에서 \(A(\frac{p}{q}, \frac{1}{2q^2}), B(\frac{P}{Q}, \frac{1}{2Q^2}), C(\frac{P}{Q},\frac{1}{2q^2} )\) 이므로, 다음이 성립한다 \[ \overline{AB}^2 = (\overline{AD} + \overline{EB})^2 + \frac{(Pq - pQ)^2 - 1}{Q^2 q^2} \]

여기서,

i. \(|Pq -pQ|> 1\) 이면, \(\overline{AB} > \overline{AD} + \overline{EB}\) 이므로, 두 원은 서로 떨어져 있다.

ii. \(|Pq -pQ|= 1\) 이면, \(\overline{AB} = \overline{AD} + \overline{EB}\) 이므로, 두 원은 접한다.

iii. \(|Pq -pQ| <1\) 일 수는 없다.

왜냐하면, \(p,q, P, Q\) 는 자연수이므로 \(Pq -pQ = 0\) 이면, \(p/q \ne P/Q\) 에 모순이기 때문이다.

위 세 가지 경우에서, 서로 겹쳐 있는 두 포드 원은 없음을 알 수 있다. ■


정리

\(x\) 좌표가 \(p/q\) 인 포드 원을 \(C[p/q]\) 라고 쓰자. 두 포드 원 \(C[b/a]\) 과 \(C[d/c]\)이 접하면, \(|ad - bc| = 1\) 이 성립한다.

증명

관찰 1 의 증명 중 ii) 로부터 알 수 있다. ■

3. Farey Series 와의 관계


메모

포드 원 (Ford Circles)2.gif


관련된 항목들


매스매티카 파일 및 계산 리소스

사전형태의 자료


리뷰, 에세이, 강의노트



관련논문

  • Athreya, Jayadev, Sneha Chaubey, Amita Malik, and Alexandru Zaharescu. “Geometry of Farey-Ford Polygons.” arXiv:1410.4908 [math], October 18, 2014. http://arxiv.org/abs/1410.4908.
  • Fractions L. R. Ford, The American Mathematical Monthly, Vol. 45, No. 9 (Nov., 1938), pp. 586-601

메타데이터

위키데이터

Spacy 패턴 목록

  • [{'LOWER': 'ford'}, {'LEMMA': 'circle'}]
원본 주소 "https://wiki.mathnt.net/index.php?title=포드_원_(Ford_Circles)&oldid=52535"