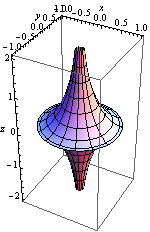
"의구 (Pseudosphere)"의 두 판 사이의 차이

수학노트
둘러보기로 가기 검색하러 가기
 
(사용자 2명의 중간 판 24개는 보이지 않습니다)
1번째 줄: 1번째 줄:
−
<h5 style="line-height: 3.428em; margin: 0px; color: rgb(34, 61, 103); font-family: 'malgun gothic',dotum,gulim,sans-serif; font-size: 1.166em; background-position: 0px 100%;">이 항목의 스프링노트 원문주소</h5>
+
==개요==
  
−
 
+
*  음의 상수곡률곡면들을 칭하는 말
 +
* [[쌍곡기하학]] 의 발전에서 중요한 역할
  
−
 
+
* [http://www.math.colostate.edu/%7Ecroke/math/summerGeom/documents/pseudosphere.pdf http://www.math.colostate.edu/~croke/math/summerGeom/documents/pseudosphere.pdf]
  
−
<h5 style="line-height: 3.428em; margin: 0px; color: rgb(34, 61, 103); font-family: 'malgun gothic',dotum,gulim,sans-serif; font-size: 1.166em; background-position: 0px 100%;">개요</h5>
+
  
−
* [http://www.math.colostate.edu/%7Ecroke/math/summerGeom/ http://www.math.colostate.edu/~croke/math/summerGeom/]<br>
+
  
−
 
+
  
−
 
+
==tractroid==
  
−
<h5 style="line-height: 3.428em; margin: 0px; color: rgb(34, 61, 103); font-family: 'malgun gothic',dotum,gulim,sans-serif; font-size: 1.166em; background-position: 0px 100%;">역사</h5>
+
* [[추적선 (tractrix)]]의 회전으로 얻어지는 곡면
 +
[[파일:10439822-추적선 (tractrix)1.gif]]
  
−
 
+
* 매개화:<math>\mathbf{x}(u,v)=(\cos (u) \text{sech}(v),\sin (u) \text{sech}(v),v-\tanh (v))</math>
  
−
* http://www.google.com/search?hl=en&tbs=tl:1&q=
+
[[파일:의구 (Pseudosphere)2.gif]]
−
* [[수학사연표 (역사)|수학사연표]]
 
−
*  
 
  
−
 
+
  
−
 
+
  
−
<h5 style="line-height: 3.428em; margin: 0px; color: rgb(34, 61, 103); font-family: 'malgun gothic',dotum,gulim,sans-serif; font-size: 1.166em; background-position: 0px 100%;">메모</h5>
+
  
−
* http://xahlee.org/surface/pseudosphere/pseudosphere.html<br>
+
==제1기본형식과 크리스토펠 기호==
  
−
 
+
* <math>E=\text{sech}^2(v)</math>, <math>F=0</math>,<math>G=\tanh ^2(v)</math>
 +
*  크리스토펠 기호:<math>\begin{array}{ll}  \Gamma _{11}^1 & 0 \\  \Gamma _{12}^1 & -\tanh (v) \\  \Gamma _{21}^1 & -\tanh (v) \\  \Gamma _{22}^1 & 0 \\  \Gamma _{11}^2 & \text{csch}(v) \text{sech}(v) \\  \Gamma _{12}^2 & 0 \\  \Gamma _{21}^2 & 0 \\  \Gamma _{22}^2 & \text{csch}(v) \text{sech}(v) \end{array}</math>
  
−
 
+
  
−
<h5 style="line-height: 3.428em; margin: 0px; color: rgb(34, 61, 103); font-family: 'malgun gothic',dotum,gulim,sans-serif; font-size: 1.166em; background-position: 0px 100%;">관련된 항목들</h5>
+
  
−
* [[상수곡률곡면과 사인-고든 방정식]]<br>
+
  
−
 
+
==역사==
 +
* 1829 - 볼리아이, 가우스, 로바체프스키가 [[쌍곡기하학]]을 발견
 +
* 1868 - 벨트라미에 의해 '의구(pseudosferiche)'라는 이름이 붙여짐
 +
* [[수학사 연표]]
 +
  
−
 
+
  
−
<h5 style="line-height: 3.428em; margin: 0px; color: rgb(34, 61, 103); font-family: 'malgun gothic',dotum,gulim,sans-serif; font-size: 1.166em; background-position: 0px 100%;">수학용어번역</h5>
+
  
−
* 단어사전 http://www.google.com/dictionary?langpair=en|ko&q=
+
==메모==
−
* 발음사전 http://www.forvo.com/search/
 
−
* [http://mathnet.kaist.ac.kr/mathnet/math_list.php?mode=list&ftype=&fstr= 대한수학회 수학 학술 용어집]<br>
 
−
**  pseudo-sphere     의구, 유사구면<br>
 
−
** http://mathnet.kaist.ac.kr/mathnet/math_list.php?mode=list&ftype=eng_term&fstr=Pseudo
 
−
* [http://www.nktech.net/science/term/term_l.jsp?l_mode=cate&s_code_cd=MA 남·북한수학용어비교]
 
−
* [http://kms.or.kr/home/kor/board/bulletin_list_subject.asp?bulletinid=%7BD6048897-56F9-43D7-8BB6-50B362D1243A%7D&boardname=%BC%F6%C7%D0%BF%EB%BE%EE%C5%E4%B7%D0%B9%E6&globalmenu=7&localmenu=4 대한수학회 수학용어한글화 게시판]
 
  
−
 
+
* http://xahlee.org/surface/pseudosphere/pseudosphere.html
 +
* http://www.math.colostate.edu/~croke/math/summerGeom/
 +
* http://danielwalsh.tumblr.com/day/2010/12/11
  
−
 
+
  
−
<h5 style="line-height: 3.428em; margin: 0px; color: rgb(34, 61, 103); font-family: 'malgun gothic',dotum,gulim,sans-serif; font-size: 1.166em; background-position: 0px 100%;">사전 형태의 자료</h5>
+
==관련된 항목들==
 +
 
 +
* [[상수곡률곡면과 사인-고든 방정식]]
 +
* [[쌍곡기하학]]
 +
 
 +
 +
 
 +
 +
 
 +
 +
 
 +
==매스매티카 파일 및 계산 리소스==
 +
 
 +
* https://docs.google.com/leaf?id=0B8XXo8Tve1cxOTIxMGRhNzAtNmY1OS00NzRlLWFkYTQtMzE2ZjlmZWM5NDdh&sort=name&layout=list&num=50
 +
 
 +
 
 +
 +
 
 +
 +
 
 +
==수학용어번역==
 +
* {{학술용어집|url=Pseudo}}
 +
*  pseudo-sphere    의구, 유사구면
 +
 
 +
 +
 
 +
==사전 형태의 자료==
  
 
* http://ko.wikipedia.org/wiki/
 
* http://ko.wikipedia.org/wiki/
 
* http://en.wikipedia.org/wiki/Pseudosphere
 
* http://en.wikipedia.org/wiki/Pseudosphere
 +
 +
 +
==리뷰, 에세이, 강의노트==
 +
* Claudio Bartocci [http://academiccommons.columbia.edu/catalog/ac:153401 Perrault's watch and Beltrami's pseudosphere: A story without a moral]
 +
 +
 +
==관련논문==
 +
* Taioli, Simone, Ruggero Gabbrielli, Stefano Simonucci, Nicola Maria Pugno, and Alfredo Iorio. “Imaginary Crystals Made Real.” arXiv:1511.07672 [cond-Mat, Physics:gr-Qc, Physics:hep-Th], November 24, 2015. http://arxiv.org/abs/1511.07672.
 +
 +
 +
[[분류:미분기하학]]
 +
[[분류:곡면]]
 +
[[분류:쌍곡기하학]]
 +
 +
==메타데이터==
 +
===위키데이터===
 +
* ID :  [https://www.wikidata.org/wiki/Q1458609 Q1458609]
 +
===Spacy 패턴 목록===
 +
* [{'LEMMA': 'pseudosphere'}]

2021년 2월 17일 (수) 05:56 기준 최신판

개요

  • 음의 상수곡률곡면들을 칭하는 말
  • 쌍곡기하학 의 발전에서 중요한 역할




tractroid

10439822-추적선 (tractrix)1.gif

  • 매개화\[\mathbf{x}(u,v)=(\cos (u) \text{sech}(v),\sin (u) \text{sech}(v),v-\tanh (v))\]

의구 (Pseudosphere)2.gif




제1기본형식과 크리스토펠 기호

  • \(E=\text{sech}^2(v)\), \(F=0\),\(G=\tanh ^2(v)\)
  • 크리스토펠 기호\[\begin{array}{ll} \Gamma _{11}^1 & 0 \\ \Gamma _{12}^1 & -\tanh (v) \\ \Gamma _{21}^1 & -\tanh (v) \\ \Gamma _{22}^1 & 0 \\ \Gamma _{11}^2 & \text{csch}(v) \text{sech}(v) \\ \Gamma _{12}^2 & 0 \\ \Gamma _{21}^2 & 0 \\ \Gamma _{22}^2 & \text{csch}(v) \text{sech}(v) \end{array}\]




역사

  • 1829 - 볼리아이, 가우스, 로바체프스키가 쌍곡기하학을 발견
  • 1868 - 벨트라미에 의해 '의구(pseudosferiche)'라는 이름이 붙여짐
  • 수학사 연표




메모


관련된 항목들




매스매티카 파일 및 계산 리소스




수학용어번역

  • Pseudo - 대한수학회 수학용어집
  • pseudo-sphere 의구, 유사구면


사전 형태의 자료


리뷰, 에세이, 강의노트


관련논문

  • Taioli, Simone, Ruggero Gabbrielli, Stefano Simonucci, Nicola Maria Pugno, and Alfredo Iorio. “Imaginary Crystals Made Real.” arXiv:1511.07672 [cond-Mat, Physics:gr-Qc, Physics:hep-Th], November 24, 2015. http://arxiv.org/abs/1511.07672.

메타데이터

위키데이터

Spacy 패턴 목록

  • [{'LEMMA': 'pseudosphere'}]
원본 주소 "https://wiki.mathnt.net/index.php?title=의구_(Pseudosphere)&oldid=52435"