"로저스 다이로그 함수 (Rogers dilogarithm)"의 두 판 사이의 차이

수학노트
둘러보기로 가기 검색하러 가기
(section '관련논문' updated)
 
(같은 사용자의 중간 판 4개는 보이지 않습니다)
1번째 줄: 1번째 줄:
 
==개요==
 
==개요==
  
−
* [[다이로그 함수(dilogarithm)]] 의 변종<br>
+
* [[다이로그 함수(dilogarithm)]] 의 변종
−
*  다양한 [[함수 다이로그 항등식(functional dilogarithm identity)]]  을 만족시킴<br>
+
*  다양한 [[함수 다이로그 항등식(functional dilogarithm identity)]]  을 만족시킴
  
 
   
 
   
10번째 줄: 10번째 줄:
 
* <math>x\in (0,1)</math>에서 로저스 다이로그 함수를 다음과 같이 정의
 
* <math>x\in (0,1)</math>에서 로저스 다이로그 함수를 다음과 같이 정의
 
:<math>L(x)=\operatorname{Li}_ 2(x)+\frac{1}{2}\log x\log (1-x)=-\frac{1}{2}\int_{0}^{x}\left(\frac{\log(1-y)}{y}+\frac{\log(y)}{1-y}\right)dy</math>
 
:<math>L(x)=\operatorname{Li}_ 2(x)+\frac{1}{2}\log x\log (1-x)=-\frac{1}{2}\int_{0}^{x}\left(\frac{\log(1-y)}{y}+\frac{\log(y)}{1-y}\right)dy</math>
−
* <math>(-\infty,0],[1,+\infty)</math>를 제외한 복소평면으로 해석적확장됨<br>
+
* <math>(-\infty,0],[1,+\infty)</math>를 제외한 복소평면으로 해석적확장됨
−
* <math>dL(y)=\frac{1}{2}[\log(y)d\log (1-y)-\log(1-y)d\log (y)]</math><br>
+
* <math>dL(y)=\frac{1}{2}[\log(y)d\log (1-y)-\log(1-y)d\log (y)]</math>
  
  
 
==함수의 그래프==
 
==함수의 그래프==
  
−
* <math>x\in (0,1)</math> 에서의 그래프<br>
+
* <math>x\in (0,1)</math> 에서의 그래프
 
[[파일:로저스 다이로그 함수 (Roger s dilogarithm)1.gif]]
 
[[파일:로저스 다이로그 함수 (Roger s dilogarithm)1.gif]]
  
−
*  함수 방정식을 이용한 확장<br>
+
*  함수 방정식을 이용한 확장
 
[[파일:로저스 다이로그 함수 (Roger s dilogarithm)2.gif]]
 
[[파일:로저스 다이로그 함수 (Roger s dilogarithm)2.gif]]
  
 
==반사공식(오일러)==
 
==반사공식(오일러)==
  
−
* <math>0\leq x \leq 1</math> 일 때:<math>L(x)+L(1-x)=L(1)</math><br>
+
* <math>0\leq x \leq 1</math> 일 때:<math>L(x)+L(1-x)=L(1)</math>
  
 
   
 
   
32번째 줄: 32번째 줄:
 
==5항 관계식==
 
==5항 관계식==
  
−
* <math>0\leq x,y\leq 1</math> 일 때, :<math>L(x)+L(1-xy)+L(y)+L(\frac{1-y}{1-xy})+L\left( \frac{1-x}{1-xy}\right)=\frac{\pi^2}{2}</math><br>
+
* <math>0\leq x,y\leq 1</math> 일 때, :<math>L(x)+L(1-xy)+L(y)+L(\frac{1-y}{1-xy})+L\left( \frac{1-x}{1-xy}\right)=\frac{\pi^2}{2}</math>
  
  
50번째 줄: 50번째 줄:
 
<math>L(\frac{-1+\sqrt{5}}{2})=\frac{\pi^2}{10}</math>
 
<math>L(\frac{-1+\sqrt{5}}{2})=\frac{\pi^2}{10}</math>
  
−
* non-unitary <math>c(2,k+2)</math> minimal models :<math>\sum_{i=1}^{[k/2]}L(\frac{\sin^2\frac{\pi}{k+2}}{\sin^2\frac{(i+1)\pi}{k+2}})=\frac{k-1}{k+2}\cdot \frac{\pi^2}{6}</math><br>
+
* non-unitary <math>c(2,k+2)</math> minimal models
 +
:<math>\sum_{i=1}^{[k/2]}L\left(\frac{\sin^2\frac{\pi}{k+2}}{\sin^2\frac{(i+1)\pi}{k+2}}\right)=\frac{k-1}{k+2}\cdot \frac{\pi^2}{6}</math>
 +
 
  
 
==역사==
 
==역사==
68번째 줄: 70번째 줄:
 
==관련된 항목들==
 
==관련된 항목들==
  
−
* [[로저스-라마누잔 항등식|로저스-라마누잔 연분수와 항등식]]<br>
+
* [[로저스-라마누잔 항등식|로저스-라마누잔 연분수와 항등식]]
  
 
   
 
   
80번째 줄: 82번째 줄:
 
* http://functions.wolfram.com/
 
* http://functions.wolfram.com/
 
* [http://dlmf.nist.gov/ NIST Digital Library of Mathematical Functions]
 
* [http://dlmf.nist.gov/ NIST Digital Library of Mathematical Functions]
−
* [http://www.research.att.com/%7Enjas/sequences/index.html The On-Line Encyclopedia of Integer Sequences]
 
−
* [http://numbers.computation.free.fr/Constants/constants.html Numbers, constants and computation]
 
−
 
 
* [[매스매티카 파일 목록]]
 
* [[매스매티카 파일 목록]]
  
 
   
 
   
−
 
−
 
−
 
−
==수학용어번역==
 
−
 
−
* http://www.google.com/dictionary?langpair=en|ko&q=
 
−
* [http://mathnet.kaist.ac.kr/mathnet/math_list.php?mode=list&ftype=&fstr= 대한수학회 수학 학술 용어집]<br>
 
−
** http://mathnet.kaist.ac.kr/mathnet/math_list.php?mode=list&ftype=eng_term&fstr=
 
−
* [http://kms.or.kr/home/kor/board/bulletin_list_subject.asp?bulletinid=%7BD6048897-56F9-43D7-8BB6-50B362D1243A%7D&boardname=%BC%F6%C7%D0%BF%EB%BE%EE%C5%E4%B7%D0%B9%E6&globalmenu=7&localmenu=4 대한수학회 수학용어한글화 게시판]
 
  
 
   
 
   
110번째 줄: 100번째 줄:
  
 
==관련논문==
 
==관련논문==
−
* [http://dx.doi.org/10.1023/A:1009709927327 Algebraic Dilogarithm Identities]<br>
+
* Tomoki Nakanishi, Rogers dilogarithms of higher degree and generalized cluster algebras, arXiv:1605.04777 [math.QA], May 16 2016, http://arxiv.org/abs/1605.04777
 +
* Hartnick, Tobias, and Andreas Ott. “Perturbations of the Spence-Abel Equation and Deformations of the Dilogarithm Function.” arXiv:1601.07109 [math], January 26, 2016. http://arxiv.org/abs/1601.07109.
 +
* [http://dx.doi.org/10.1023/A:1009709927327 Algebraic Dilogarithm Identities]
 
** Basil Gordon  and Richard J. Mcintosh, 1997
 
** Basil Gordon  and Richard J. Mcintosh, 1997
−
* [http://dx.doi.org/10.1143/PTPS.118.61 Dilogarithm identities]<br>
+
* [http://dx.doi.org/10.1143/PTPS.118.61 Dilogarithm identities]
 
** Anatol N. Kirillov,Prog.Theor.Phys.Suppl.118:61-142, 1995
 
** Anatol N. Kirillov,Prog.Theor.Phys.Suppl.118:61-142, 1995
−
* [http://dx.doi.org/10.1007/BF01840426 Identities for the Rogers dilogarithm function connected with simple Lie algebras]<br>
+
* [http://dx.doi.org/10.1007/BF01840426 Identities for the Rogers dilogarithm function connected with simple Lie algebras]
 
** A. N. Kirillov, 1989
 
** A. N. Kirillov, 1989
−
* http://www.jstor.org/action/doBasicSearch?Query=
 
−
* http://dx.doi.org/10.1023/A:1009709927327
 
−
 
−
 
−
 
−
 
  
 
==관련도서==
 
==관련도서==
−
*  Harold Scott Macdonald Coxeter [http://books.google.com/books?id=beTjmcibCH8C The beauty of geometry: twelve essays]<br>
+
*  Harold Scott Macdonald Coxeter [http://books.google.com/books?id=beTjmcibCH8C The beauty of geometry: twelve essays]
 
** chapter 1
 
** chapter 1
 
[[분류:다이로그]]
 
[[분류:다이로그]]
 +
[[분류:특수함수]]

2016년 5월 18일 (수) 00:14 기준 최신판

개요


정의

  • \(x\in (0,1)\)에서 로저스 다이로그 함수를 다음과 같이 정의

\[L(x)=\operatorname{Li}_ 2(x)+\frac{1}{2}\log x\log (1-x)=-\frac{1}{2}\int_{0}^{x}\left(\frac{\log(1-y)}{y}+\frac{\log(y)}{1-y}\right)dy\]

  • \((-\infty,0],[1,+\infty)\)를 제외한 복소평면으로 해석적확장됨
  • \(dL(y)=\frac{1}{2}[\log(y)d\log (1-y)-\log(1-y)d\log (y)]\)


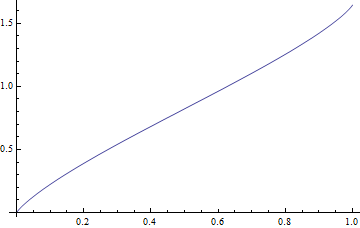
함수의 그래프

  • \(x\in (0,1)\) 에서의 그래프

로저스 다이로그 함수 (Roger s dilogarithm)1.gif

  • 함수 방정식을 이용한 확장

로저스 다이로그 함수 (Roger s dilogarithm)2.gif

반사공식(오일러)

  • \(0\leq x \leq 1\) 일 때\[L(x)+L(1-x)=L(1)\]



5항 관계식

  • \(0\leq x,y\leq 1\) 일 때, \[L(x)+L(1-xy)+L(y)+L(\frac{1-y}{1-xy})+L\left( \frac{1-x}{1-xy}\right)=\frac{\pi^2}{2}\]


special values

\(L(0)=0\)

\(L(1)=\frac{\pi^2}{6}\)

\(L(-1)=-\frac{\pi^2}{12}\)

\(L(\frac{1}{2})=\frac{\pi^2}{12}\)

\(L(\frac{3-\sqrt{5}}{2})=\frac{\pi^2}{15}\)

\(L(\frac{-1+\sqrt{5}}{2})=\frac{\pi^2}{10}\)

  • non-unitary \(c(2,k+2)\) minimal models

\[\sum_{i=1}^{[k/2]}L\left(\frac{\sin^2\frac{\pi}{k+2}}{\sin^2\frac{(i+1)\pi}{k+2}}\right)=\frac{k-1}{k+2}\cdot \frac{\pi^2}{6}\]


역사



메모

관련된 항목들



매스매티카 파일 및 계산 리소스




사전 형태의 자료



관련논문

관련도서

원본 주소 "https://wiki.mathnt.net/index.php?title=로저스_다이로그_함수_(Rogers_dilogarithm)&oldid=32748"