Nested radicals

개요

• 황금비\[\sqrt{1+\sqrt{1+\sqrt{1+\sqrt{1+\sqrt{1+\cdots}}}}}=\varphi=\frac{1+\sqrt5}{2}=1.61803398874989\cdots\]
  
• 비에타의 공식\[\frac{2}{\pi}=\frac{\sqrt{2}}{2}\frac{\sqrt{2+\sqrt{2}}}{2} \frac{\sqrt{2+\sqrt{2+\sqrt{2}}}}{2} \frac{\sqrt{2+\sqrt{2+\sqrt{2+\sqrt{2}}}}}{2} \frac{\sqrt{2+\sqrt{2+\sqrt{2+\sqrt{2+\sqrt{2}}}}}}{2} \frac{\sqrt{2+\sqrt{2+\sqrt{2+\sqrt{2+\sqrt{2+\sqrt{2}}}}}}}{2}\cdots\]
  
• nested radical 상수\[\sqrt{1+\sqrt{2+\sqrt{3+\sqrt{4+\sqrt{5+\sqrt{6+\cdots}}}}}}=1.75793275661800453270881963821820816125\cdots\]
  
• 삼각함수의 값\[\cos \frac{\pi}{32}=\cos\frac{\pi}{2^5}= \frac{\sqrt{2+\sqrt{2+\sqrt{2+\sqrt{2}}}}}{2}\]\[\cos \frac{\pi}{64}=\cos\frac{\pi}{2^6}= \frac{\sqrt{2+\sqrt{2+\sqrt{2+\sqrt{2+\sqrt{2}}}}}}{2}\]
  

 

 

라마누잔이 제시한 문제

• \(\sqrt{1+2\sqrt{1+3\sqrt{1+4\sqrt{1+5\sqrt{1+6\cdots}}}}} = 3\)
  
• 다음 수열의 극한\[1,\sqrt{1+2 },\sqrt{1+2 \sqrt{1+3 }},\sqrt{1+2 \sqrt{1+3 \sqrt{1+4 }}},\sqrt{1+2 \sqrt{1+3 \sqrt{1+4 \sqrt{1+5 }}}},\sqrt{1+2 \sqrt{1+3 \sqrt{1+4 \sqrt{1+5 \sqrt{1+6 }}}}}, \cdots\]
  

 

 

증명

먼저 수렴성을 증명하자. 다음과 같이 정의된 수열 

\(1,\sqrt{1+2 },\sqrt{1+2 \sqrt{1+3 }},\sqrt{1+2 \sqrt{1+3 \sqrt{1+4 }}},\sqrt{1+2 \sqrt{1+3 \sqrt{1+4 \sqrt{1+5 }}}},\sqrt{1+2 \sqrt{1+3 \sqrt{1+4 \sqrt{1+5 \sqrt{1+6 }}}}}, \cdots\) 은 위로 유계이다.

 

\(\sqrt{1+2 \sqrt{1+3\sqrt{1+\cdots+ (n-1)\sqrt{1+n} }}} \leq \sqrt{1+2 \sqrt{1+3\sqrt{1+\cdots+ (n-1)\sqrt{1+n(n+2)} }}}=3\)

 

\(n=\sqrt{1+(n-1)(n+1)}\)을 이용

\(\begin{eqnarray*}3 &=& \sqrt{1+2\cdot4}\\ &=& \sqrt{1+2\sqrt{1+3\cdot5}}\\ &=& \sqrt{1+2\sqrt{1+3\sqrt{1+4\cdot6}}}\\ &=& \cdots\end{eqnarray*}\)

 

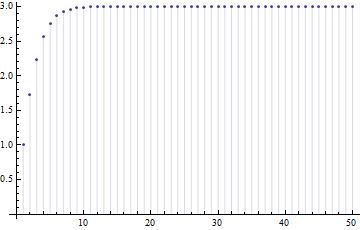
수열의 크기 변화를 나타내는 그래프

\(1,\sqrt{1+2 },\sqrt{1+2 \sqrt{1+3 }},\sqrt{1+2 \sqrt{1+3 \sqrt{1+4 }}},\sqrt{1+2 \sqrt{1+3 \sqrt{1+4 \sqrt{1+5 }}}},\sqrt{1+2 \sqrt{1+3 \sqrt{1+4 \sqrt{1+5 \sqrt{1+6 }}}}}, \cdots\)

 

매쓰매티카 코드

1. f[n_][x_]:=Sqrt[1+n*x]
   a[1][x_]:=x
   a[n_][x_]:=Composition[a[n-1],f[n]][x]
   Table[a[n][x],{n,1,6}]
   DiscretePlot[a[n][1],{n,1,50}]

• 결과

\(\left\{x,\sqrt{1+2 x},\sqrt{1+2 \sqrt{1+3 x}},\sqrt{1+2 \sqrt{1+3 \sqrt{1+4 x}}},\sqrt{1+2 \sqrt{1+3 \sqrt{1+4 \sqrt{1+5 x}}}},\sqrt{1+2 \sqrt{1+3 \sqrt{1+4 \sqrt{1+5 \sqrt{1+6 x}}}}}\right\}\)

 

함수방정식

• $f(x)=\sqrt{1+x \sqrt{1+(x+1) \sqrt{1+(x+2) \sqrt{\cdots}}}}$
• $[f(x)]^2=1+xf(x+1), f(x)\ge 0$
• $f(x)=x+1$
• Functional Equations and and How to Solve Them Section 3.8 Functional equations and nested radicals

 

메모

• http://www.dgp.toronto.edu/~mjmcguff/math/nestedRadicals.pdf
• http://fluxionsdividebyzero.com/p1/math/calculus/number/cr/sr_nroots.pdf

 

 

관련된 항목들

• 연분수

 

 

관련도서

• Ramanujan, S. Collected Papers of Srinivasa Ramanujan (Ed. G. H. Hardy, P. V. S. Aiyar, and B. M. Wilson). Providence, RI: Amer. Math. Soc., 2000.
• Functional Equations and and How to Solve Them
  • section 3.8

 

 

관련논문

• Ramanujan, S. Question No. 298. J. Indian Math. Soc. 1911.

 

 

사전형태의 참고자료

• http://en.wikipedia.org/wiki/Nested_radical
• http://mathworld.wolfram.com/NestedRadical.html

 

 

블로그

• Ramanujan's infinitely nested radicals problem[1]
  
  • New Start, Ens! , 2009-1-16