유클리드 평면의 테셀레이션
둘러보기로 가기
검색하러 가기
개요
- 삼각형에 의한 유클리드 평면의 테셀레이션은 세 가지 경우 : (4 4 2), (3 3 3), (6 3 2)
- (6 3 2)란 삼각형의 세 각이 각각 \(\frac{\pi}{6},\frac{\pi}{3},\frac{\pi}{2}=\pi\)로 주어지는 경우
- \(\frac{\pi}{6}+\frac{\pi}{3}+\frac{\pi}{2}=\pi\) 가 되어 삼각형이 세 각의 합이 180도가 된다
- 평면의 곡률이 0 이기 때문에 나타나는 현상이다.
삼각형에 의한 테셀레이션
디오판투스 방정식
- \((v_1,v_2,v_3)\)-삼각형이 테셀레이션이 되려면 다음의 디오판투스 방정식을 만족해야 한다
\[\frac{1}{v_{1}}+\frac{1}{v_{2}}+\frac{1}{v_{3}}=1\] 해는 다음과 같다 \[(v_1,v_2,v_3)=(3,3,3), (2,3,6), (2,4,4),\]
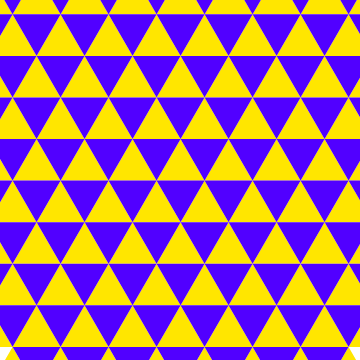
(3,3,3) 삼각형
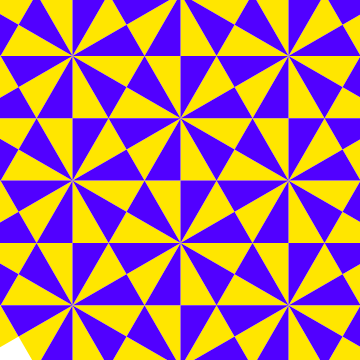
(2,3,6) 삼각형
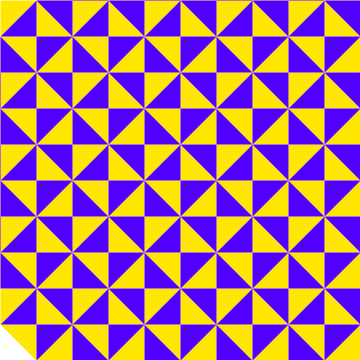
(2,4,4) 삼각형
관련된 항목들
매스매티카 파일 및 계산 리소스
- https://docs.google.com/file/d/0B8XXo8Tve1cxcEtiams4cTRHTDA/edit
- http://mathematica.stackexchange.com/questions/45903/coloring-a-honeycomb-structure?atw=1
- http://mathematica.stackexchange.com/questions/61834/how-to-create-an-hexagonal-lattice-structure
- http://mathematica.stackexchange.com/questions/89138/drawing-a-trihexagonal-tiling