"푸앵카레 상반평면 모델"의 두 판 사이의 차이
Pythagoras0 (토론 | 기여) (→측지선) |
Pythagoras0 (토론 | 기여) |
||
| (같은 사용자의 중간 판 18개는 보이지 않습니다) | |||
| 1번째 줄: | 1번째 줄: | ||
| − | |||
==개요== | ==개요== | ||
| 18번째 줄: | 17번째 줄: | ||
==제1기본형식== | ==제1기본형식== | ||
| − | * 리만 메트릭 | + | * 리만 메트릭:<math>ds^2=\frac{dx^2+dy^2}{y^2}=\frac{dzd\overline{z}}{y^2}</math> |
* <math>E=1/y^2</math> | * <math>E=1/y^2</math> | ||
| 24번째 줄: | 23번째 줄: | ||
* <math>G=1/y^2</math> | * <math>G=1/y^2</math> | ||
| − | * 면적소 | + | * 면적소:<math>dA=\frac{dx\,dy}{y^2}</math> |
| − | * 두 점 사이의 거리 | + | * 두 점 사이의 거리 |
| + | :<math>\rho(z_ 1,z_ 2)=2\tanh^{-1}\frac{|z_ 1-z_ 2|}{|z_ 1-\overline{z_ 2}|}</math> | ||
| + | :<math> | ||
| + | \cosh \rho(z_ 1,z_ 2)=1+\frac{|z_1-z_2|^2}{2y_1y_2}= | ||
| + | \frac{\left(x_1-x_2\right)^2+y_1^2+y_2^2}{2 y_1 y_2} | ||
| + | </math> | ||
| + | |||
| − | |||
| − | |||
==크리스토펠 기호== | ==크리스토펠 기호== | ||
| − | * [[크리스토펠 기호]] | + | * [[크리스토펠 기호]]:<math>\begin{array}{ll} \Gamma _ {11}^1 & 0 \\ \Gamma _ {12}^1 & -\frac{1}{y} \\ \Gamma _ {21}^1 & -\frac{1}{y} \\ \Gamma _ {22}^1 & 0 \\ \Gamma _ {11}^2 & \frac{1}{y} \\ \Gamma _ {12}^2 & 0 \\ \Gamma _ {21}^2 & 0 \\ \Gamma _ {22}^2 & -\frac{1}{y} \end{array}</math> |
| + | * 등장변환군(isometry group) | ||
| + | :<math>\operatorname{PSL}(2,\mathbb{R})\rtimes \mathbb{Z}/2\mathbb{Z}</math> | ||
| + | * [[가우스 곡률|가우스곡률]] 은 -1 이다 | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
==라플라시안== | ==라플라시안== | ||
| − | * [[라플라시안(Laplacian)|라플라시안]] | + | * [[라플라시안(Laplacian)|라플라시안]]:<math>\Delta f=y^2(\frac{\partial^2 f}{\partial x^2}+\frac{\partial^2 f}{\partial y^2})</math> |
| 52번째 줄: | 53번째 줄: | ||
==측지선== | ==측지선== | ||
| − | * [[측지선]]이 만족시키는 미분방정식은 다음과 같다 | + | * [[측지선]]이 만족시키는 미분방정식은 다음과 같다 |
| − | :<math>\frac{d^2 x }{dt^2} + \Gamma^{1}_{1 2 }\frac{dx }{dt}\frac{dy }{dt} +\Gamma^{1}_{2 1 }\frac{dx }{dt}\frac{dy }{dt}= 0 | + | :<math>\left\{ \begin{array}{c} |
| − | + | \frac{d^2 x }{dt^2} + \Gamma^{1}_{1 2 }\frac{dx }{dt}\frac{dy }{dt} +\Gamma^{1}_{2 1 }\frac{dx }{dt}\frac{dy }{dt}= 0 \\ | |
| − | * 다시 쓰면 다음과 같다 | + | \frac{d^2 y }{dt^2} + \Gamma^{2}_{1 1 }\frac{dx }{dt}\frac{dx }{dt} +\Gamma^{2}_{2 2 }\frac{dy }{dt}\frac{dy }{dt} = 0 |
| − | * 미분방정식의 해는 적당한 상수 a,b,c,r 에 대하여 다음과 같이 주어진다< | + | \end{array} \right. </math> |
| + | * 다시 쓰면 다음과 같다 | ||
| + | :<math> \left\{ \begin{array}{c} \ddot{x}y-2\dot{x}\dot{y}=0 \\ \ddot{y}y+(\dot{x})^2-(\dot{y})^2=0 \end{array} \right. </math> | ||
| + | * 미분방정식의 해는 적당한 상수 a,b,c,r 에 대하여 다음과 같이 주어진다 | ||
| + | ** <math>(x(t),y(t))</math>로 매개화된 실직선에 수직인 반원 :<math>\left\{ \begin{array}{c} | ||
| + | x(t)=a+b\tanh(rt+c) \\ | ||
| + | y(t)=b\operatorname{sech}(rt+c) | ||
| + | \end{array} \right. </math> <math>\tanh ^{2}x=1-\operatorname{sech}^{2}x</math> [[쌍곡함수]]) | ||
| + | ** <math>(x(t),y(t))</math>로 매개화된 y-축과 평행한 직선 :<math>\left\{ \begin{array}{c} | ||
| + | x(t)=a \\ | ||
| + | y(t)=be^{rt+c} | ||
| + | \end{array} \right. </math> | ||
* http://cktalon.wordpress.com/2010/10/22/geodesics-poincare-half-plane/ | * http://cktalon.wordpress.com/2010/10/22/geodesics-poincare-half-plane/ | ||
| + | |||
| + | |||
==리만 텐서== | ==리만 텐서== | ||
| 68번째 줄: | 82번째 줄: | ||
==쌍곡삼각형의 넓이== | ==쌍곡삼각형의 넓이== | ||
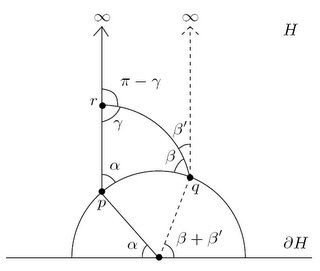
| − | + | [[파일:Hyperbolic triangle.jpg]] | |
| − | + | * 삼각형<math>D=pq\infty</math>의 넓이:<math>x(P)</math> 를 점 <math>P</math>의 <math>x</math>좌표라 하고, <math>x(p)=a</math>, <math>x(q)=b</math>라 두자. :<math>A(D)=\int\int_{D}\frac{dx\,dy}{y^2}=\int_{a}^{b}\int_{\sqrt{1-x^2}}^{\infty}\frac{dy\,dx}{y^2}=\int_{a}^{b}\frac{1}{\sqrt{1-x^2}}\,dx=-\int_{\pi-\alpha}^{\beta+\beta'}\,d\theta=\pi-\alpha-\beta-\beta'</math>:<math>x=\cos \theta</math>로 치환, <math>a=\cos (\pi-\alpha)</math>, <math>b=\cos (\beta+\beta')</math>을 사용하였음 | |
| + | * 삼각형 <math>D'=rq\infty</math>의 넓이 위에서 얻은 결과를 적용할 수 있다 :<math>A(D')=\pi-(\pi-\gamma)-\beta'=\gamma-\beta'</math> | ||
| − | + | ||
| − | |||
| − | |||
| − | |||
(정리) | (정리) | ||
| − | 세 각이 <math>\alpha, \beta, \gamma</math>인 쌍곡삼각형 <math>\Delta</math>의 넓이는 <math>\pi - \alpha- \beta- \gamma</math> 로 주어진다 | + | 세 각이 <math>\alpha, \beta, \gamma</math>인 쌍곡삼각형 <math>\Delta</math>의 넓이는 <math>\pi - \alpha- \beta- \gamma</math> 로 주어진다. |
| − | + | ||
(증명) | (증명) | ||
| 87번째 줄: | 99번째 줄: | ||
<math>A(\Delta)=A(D)-A(D')=\pi-\alpha-\beta-\beta'-(\gamma-\beta')=\pi - \alpha- \beta- \gamma</math> ■ | <math>A(\Delta)=A(D)-A(D')=\pi-\alpha-\beta-\beta'-(\gamma-\beta')=\pi - \alpha- \beta- \gamma</math> ■ | ||
| − | + | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | * [[가우스-보네 정리]]로도 같은 결과를 얻을 수 있으며, 더 일반적인 곡면에 적용가능하다 | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
==역사== | ==역사== | ||
| − | * [[ | + | * [[수학사 연표]] |
| − | + | ||
| − | + | ||
==메모== | ==메모== | ||
| 143번째 줄: | 118번째 줄: | ||
* [http://www.math.sunysb.edu/%7Emalkoun/hyperbolic.pdf http://www.math.sunysb.edu/~malkoun/hyperbolic.pdf] | * [http://www.math.sunysb.edu/%7Emalkoun/hyperbolic.pdf http://www.math.sunysb.edu/~malkoun/hyperbolic.pdf] | ||
| − | + | ||
| − | + | ||
==관련된 항목들== | ==관련된 항목들== | ||
| − | + | * [[2차원 쌍곡기하학의 테셀레이션]] | |
| − | * [[로바체프스키 | + | * [[케일리 뫼비우스 변환]] |
| + | * [[로바체프스키 함수]] | ||
* [[삼각함수와 쌍곡함수의 맥클로린 급수]] | * [[삼각함수와 쌍곡함수의 맥클로린 급수]] | ||
| − | * [[ | + | * [[이와사와 분해 (Iwasawa decomposition)]] |
| + | |||
| − | + | ||
| − | |||
| − | |||
==매스매티카 파일 및 계산 리소스== | ==매스매티카 파일 및 계산 리소스== | ||
* https://docs.google.com/file/d/0B8XXo8Tve1cxOGNiYzY2ODctYThkZC00Mzg1LWI4OGQtMWZiMmUyYmIyMjBi/edit | * https://docs.google.com/file/d/0B8XXo8Tve1cxOGNiYzY2ODctYThkZC00Mzg1LWI4OGQtMWZiMmUyYmIyMjBi/edit | ||
| − | * http://www.wolframalpha.com/input/?i= | + | * http://www.wolframalpha.com/input/?i=upper-half+plane |
| − | + | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
==수학용어번역== | ==수학용어번역== | ||
| + | * {{학술용어집|url=isometry}} | ||
| + | |||
| − | + | ==사전 형태의 자료== | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | ==사전 | ||
* http://ko.wikipedia.org/wiki/ | * http://ko.wikipedia.org/wiki/ | ||
| − | * | + | * http://en.wikipedia.org/wiki/Poincaré_half-plane_model |
| − | |||
| − | |||
| − | + | [[분류:미분기하학]] | |
| + | [[분류:곡면]] | ||
| + | [[분류:쌍곡기하학]] | ||
| − | == | + | == 메타데이터 == |
| − | * | + | ==메타데이터== |
| − | * | + | ===위키데이터=== |
| + | * ID : [https://www.wikidata.org/wiki/Q2534886 Q2534886] | ||
| + | ===Spacy 패턴 목록=== | ||
| + | * [{'LOWER': 'poincaré'}, {'LOWER': 'half'}, {'OP': '*'}, {'LOWER': 'plane'}, {'LEMMA': 'model'}] | ||
| + | * [{'LOWER': 'poincare'}, {'LOWER': 'half'}, {'OP': '*'}, {'LOWER': 'plane'}, {'LEMMA': 'model'}] | ||
2021년 2월 17일 (수) 06:07 기준 최신판
개요
- 쌍곡기하학의 모델
정의
- \(\mathbb{H}^2=\{z=x+iy\in\mathbb{C}|y>0\}\)
제1기본형식
- 리만 메트릭\[ds^2=\frac{dx^2+dy^2}{y^2}=\frac{dzd\overline{z}}{y^2}\]
- \(E=1/y^2\)
- \(F=0\)
- \(G=1/y^2\)
- 면적소\[dA=\frac{dx\,dy}{y^2}\]
- 두 점 사이의 거리
\[\rho(z_ 1,z_ 2)=2\tanh^{-1}\frac{|z_ 1-z_ 2|}{|z_ 1-\overline{z_ 2}|}\] \[ \cosh \rho(z_ 1,z_ 2)=1+\frac{|z_1-z_2|^2}{2y_1y_2}= \frac{\left(x_1-x_2\right)^2+y_1^2+y_2^2}{2 y_1 y_2} \]
크리스토펠 기호
- 크리스토펠 기호\[\begin{array}{ll} \Gamma _ {11}^1 & 0 \\ \Gamma _ {12}^1 & -\frac{1}{y} \\ \Gamma _ {21}^1 & -\frac{1}{y} \\ \Gamma _ {22}^1 & 0 \\ \Gamma _ {11}^2 & \frac{1}{y} \\ \Gamma _ {12}^2 & 0 \\ \Gamma _ {21}^2 & 0 \\ \Gamma _ {22}^2 & -\frac{1}{y} \end{array}\]
- 등장변환군(isometry group)
\[\operatorname{PSL}(2,\mathbb{R})\rtimes \mathbb{Z}/2\mathbb{Z}\]
- 가우스곡률 은 -1 이다
라플라시안
- 라플라시안\[\Delta f=y^2(\frac{\partial^2 f}{\partial x^2}+\frac{\partial^2 f}{\partial y^2})\]
측지선
- 측지선이 만족시키는 미분방정식은 다음과 같다
\[\left\{ \begin{array}{c} \frac{d^2 x }{dt^2} + \Gamma^{1}_{1 2 }\frac{dx }{dt}\frac{dy }{dt} +\Gamma^{1}_{2 1 }\frac{dx }{dt}\frac{dy }{dt}= 0 \\ \frac{d^2 y }{dt^2} + \Gamma^{2}_{1 1 }\frac{dx }{dt}\frac{dx }{dt} +\Gamma^{2}_{2 2 }\frac{dy }{dt}\frac{dy }{dt} = 0 \end{array} \right. \]
- 다시 쓰면 다음과 같다
\[ \left\{ \begin{array}{c} \ddot{x}y-2\dot{x}\dot{y}=0 \\ \ddot{y}y+(\dot{x})^2-(\dot{y})^2=0 \end{array} \right. \]
- 미분방정식의 해는 적당한 상수 a,b,c,r 에 대하여 다음과 같이 주어진다
- \((x(t),y(t))\)로 매개화된 실직선에 수직인 반원 \[\left\{ \begin{array}{c} x(t)=a+b\tanh(rt+c) \\ y(t)=b\operatorname{sech}(rt+c) \end{array} \right. \] \(\tanh ^{2}x=1-\operatorname{sech}^{2}x\) 쌍곡함수)
- \((x(t),y(t))\)로 매개화된 y-축과 평행한 직선 \[\left\{ \begin{array}{c} x(t)=a \\ y(t)=be^{rt+c} \end{array} \right. \]
- http://cktalon.wordpress.com/2010/10/22/geodesics-poincare-half-plane/
리만 텐서
\(\begin{array}{ll} \begin{array}{ll} R_ {111}^1 & 0 \\ R_ {112}^1 & 0 \end{array} & \begin{array}{ll} R_ {121}^1 & 0 \\ R_ {122}^1 & 0 \end{array} \\ \begin{array}{ll} R_ {211}^1 & 0 \\ R_ {212}^1 & -\frac{1}{y^2} \end{array} & \begin{array}{ll} R_ {221}^1 & \frac{1}{y^2} \\ R_ {222}^1 & 0 \end{array} \\ \begin{array}{ll} R_ {111}^2 & 0 \\ R_ {112}^2 & \frac{1}{y^2} \end{array} & \begin{array}{ll} R_ {121}^2 & -\frac{1}{y^2} \\ R_ {122}^2 & 0 \end{array} \\ \begin{array}{ll} R_ {211}^2 & 0 \\ R_ {212}^2 & 0 \end{array} & \begin{array}{ll} R_ {221}^2 & 0 \\ R_ {222}^2 & 0 \end{array} \end{array}\)
쌍곡삼각형의 넓이
- 삼각형\(D=pq\infty\)의 넓이\[x(P)\] 를 점 \(P\)의 \(x\)좌표라 하고, \(x(p)=a\), \(x(q)=b\)라 두자. \[A(D)=\int\int_{D}\frac{dx\,dy}{y^2}=\int_{a}^{b}\int_{\sqrt{1-x^2}}^{\infty}\frac{dy\,dx}{y^2}=\int_{a}^{b}\frac{1}{\sqrt{1-x^2}}\,dx=-\int_{\pi-\alpha}^{\beta+\beta'}\,d\theta=\pi-\alpha-\beta-\beta'\]\[x=\cos \theta\]로 치환, \(a=\cos (\pi-\alpha)\), \(b=\cos (\beta+\beta')\)을 사용하였음
- 삼각형 \(D'=rq\infty\)의 넓이 위에서 얻은 결과를 적용할 수 있다 \[A(D')=\pi-(\pi-\gamma)-\beta'=\gamma-\beta'\]
(정리)
세 각이 \(\alpha, \beta, \gamma\)인 쌍곡삼각형 \(\Delta\)의 넓이는 \(\pi - \alpha- \beta- \gamma\) 로 주어진다.
(증명)
\(A(\Delta)=A(D)-A(D')=\pi-\alpha-\beta-\beta'-(\gamma-\beta')=\pi - \alpha- \beta- \gamma\) ■
- 가우스-보네 정리로도 같은 결과를 얻을 수 있으며, 더 일반적인 곡면에 적용가능하다
역사
메모
관련된 항목들
매스매티카 파일 및 계산 리소스
- https://docs.google.com/file/d/0B8XXo8Tve1cxOGNiYzY2ODctYThkZC00Mzg1LWI4OGQtMWZiMmUyYmIyMjBi/edit
- http://www.wolframalpha.com/input/?i=upper-half+plane
수학용어번역
- isometry - 대한수학회 수학용어집
사전 형태의 자료
메타데이터
메타데이터
위키데이터
- ID : Q2534886
Spacy 패턴 목록
- [{'LOWER': 'poincaré'}, {'LOWER': 'half'}, {'OP': '*'}, {'LOWER': 'plane'}, {'LEMMA': 'model'}]
- [{'LOWER': 'poincare'}, {'LOWER': 'half'}, {'OP': '*'}, {'LOWER': 'plane'}, {'LEMMA': 'model'}]