"정칠각형"의 두 판 사이의 차이
Pythagoras0 (토론 | 기여) |
Pythagoras0 (토론 | 기여) |
||
| (같은 사용자의 중간 판 21개는 보이지 않습니다) | |||
| 1번째 줄: | 1번째 줄: | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
==개요== | ==개요== | ||
| + | * 정칠각형에 관련된 여러 가지 수학적 주제의 이해 | ||
| − | + | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | ==정칠각형 꼭지점의 평면좌표== | |
| − | + | * 정칠각형이 단위원에 내접하고 있고, 한 점의 좌표가 <math>(1,0)</math>으로 주어진 경우 | |
| − | + | * 방정식 <math>z^6 + z^5 + z^4 + z^3 + z^2 + z + 1 = 0</math>은 다음과 같은 순서로 풀수 있음. | |
| − | <math>z^2 | + | * 양변을 <math>z^3</math>으로 나누면, <math>z^3+z^2+z+1+\frac{1}{z}+\frac{1}{z^2}+\frac{1}{z^3}=0</math> 을 얻게됨. |
| − | + | * <math>y=z+\frac{1}{z}</math> 로 치환하면, 원래의 방정식에서 다음 식을 얻을 수 있음. | |
| − | <math>z=\frac{ | + | :<math>z^3+z^2+z+1+\frac{1}{z}+\frac{1}{z^2}+\frac{1}{z^3}=(z+\frac{1}{z})^3+(z+\frac{1}{z})^2-2(z+\frac{1}{z})-1=y^3+y^2-2y-1=0</math> |
| − | + | [[3차 방정식의 근의 공식]]을 이용하여 방정식 <math>y^3+y^2-2y-1=0</math>을 풀면, 다음을 얻는다 | |
| − | + | :<math>y_1= \frac{1}{3} \left(-1+\frac{7^{2/3}}{\sqrt[3]{\frac{1}{2} \left(1+3 i \sqrt{3}\right)}}+\sqrt[3]{\frac{7}{2} \left(1+3 i \sqrt{3}\right)}\right)(=2\cos\frac{2\pi}{7})</math> | |
| − | + | :<math>y_2=-\frac{1}{3}-\frac{7^{2/3} \left(1+i \sqrt{3}\right)}{3\ 2^{2/3} \sqrt[3]{1+3 i \sqrt{3}}}+\frac{1}{6} i \sqrt[3]{\frac{7}{2} \left(1+3 i \sqrt{3}\right)} \left(\sqrt{3}+i\right)(=2\cos\frac{4\pi}{7})</math> | |
| − | + | :<math>y_3=-\frac{1}{3}-\frac{1}{6} \left(1+i \sqrt{3}\right) \sqrt[3]{\frac{7}{2} \left(1+3 i \sqrt{3}\right)}+\frac{i 7^{2/3} \left(\sqrt{3}+i\right)}{3\ 2^{2/3} \sqrt[3]{1+3 i \sqrt{3}}}(=2\cos\frac{6\pi}{7})</math> | |
| − | + | 이제 <math>z^2-yz+1=0</math>로부터 <math>z=\frac{y+\sqrt{y^2-4}}{2}</math> | |
| − | + | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
==정칠각형의 대각선의 길이== | ==정칠각형의 대각선의 길이== | ||
| − | * 한 변의 길이가 1인 정칠각형의 대각선의 | + | * 한 변의 길이가 1인 정칠각형의 대각선의 길이 <math>r_i</math>는 <math>i=0,1,\cdots,5</math>에 대하여 다음과 같이 주어짐 :<math>r_i=\frac{\sin \frac{(i+1)\pi}{7}}{\sin \frac{\pi}{7}}</math> * 이 때 <math>r_0=1</math>, <math>r_5=1</math>[[파일:6782509-heptagon.png]] |
| − | * 제2종 [[체비셰프 다항식]] | + | * 제2종 [[체비셰프 다항식]] |
| − | * 대각선이 만족시키는 다양한 항등식 | + | * 대각선이 만족시키는 다양한 항등식:<math>r_hr_k=r_{h-k}+r_{h-k+2}+\cdots+r_{h+k}, 0\leq k\leq h\leq 2</math>:<math>r_i^2=1+r_{i-1}r_{i+1}, 1\leq i \leq 4</math>:<math>r_0r_0=r_0</math>:<math>r_1r_0=r_1</math>:<math>r_1r_1=r_0+r_2</math>:<math>r_2r_0=r_2</math>:<math>r_2r_1=r_1+r_3</math>:<math>r_2r_2=r_0+r_2+r_4</math> |
* <math>r_1</math>은 <math>x^3-x^2-2x+1=0</math> 의 해이다 http://www.wolframalpha.com/input/?i=+%28sin%282pi%2F7%29%2Fsin%28pi%2F7%29%29 | * <math>r_1</math>은 <math>x^3-x^2-2x+1=0</math> 의 해이다 http://www.wolframalpha.com/input/?i=+%28sin%282pi%2F7%29%2Fsin%28pi%2F7%29%29 | ||
| 75번째 줄: | 40번째 줄: | ||
<math>r_2r_2=r_0+r_2+r_4=r_0+r_2+r_1</math> ■ | <math>r_2r_2=r_0+r_2+r_4=r_0+r_2+r_1</math> ■ | ||
| − | + | ||
| − | + | ||
| − | + | ||
==다이로그 항등식== | ==다이로그 항등식== | ||
| − | * [[다이로그 항등식 (dilogarithm identities)]] | + | * [[다이로그 항등식 (dilogarithm identities)]]:<math>\alpha=\frac{\sec\frac{2\pi}{7}}{2}=0.80194\cdots</math>:<math>\beta=\frac{\sec\frac{\pi}{7}}{2}=0.554958\cdots</math>:<math>\gamma=2\cos\frac{3\pi}{7}=0.445042\cdots</math>:<math>7L(\alpha^2)-7L(\alpha)+L(1)=0</math>:<math>7L(\beta^2)+14L(\beta)-10L(1)=0</math>:<math>7L(\gamma^2)+14L(\gamma)-8L(1)=0</math>:<math>\sum_{i=1}^{5}L\left(\frac{\sin^2\frac{\pi}{7}}{\sin^2\frac{(i+1)\pi}{7}}\right)=\frac{5\pi^2}{14}</math> |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | * | + | * 방정식 <math>x^3+2x^2-x-1=0</math> 의 해 <math>\alpha, -\beta, -\gamma^{-1}</math> |
| − | + | * 방정식 <math>x^3+x^2-2x-1=0</math>의 해 <math>a,b,c</math> | |
| − | + | <math>a=2\cos\frac{2\pi}{7}=\alpha^{-1}=1.24698\cdots</math>, <math>b=2\cos\frac{4\pi}{7}=-\gamma=-0.445042\cdots</math>,<math>c=2\cos\frac{6\pi}{7}=-\beta^{-1}=-1.80194\cdots</math> | |
| − | == | + | * k=3인 경우의 [[앤드류스-고든 항등식(Andrews-Gordon identity)]]:<math>1-x_{1}=x_{1}^{4}x_{2}^{2}</math>:<math>1-x_{2}=x_{1}^{2}x_{2}^{2}</math>를 풀면,:<math>x_{2}=1-\frac{1-x_{1}}{x_{1}^{2}}=\frac{x_{1}^{2}+x_{1}-1}{x_{1}^{2}}</math>:<math>1-x_{1}=(x_{1}^{2}+x_{1}-1)^{2}</math> 따라서 <math>x_1</math>은 <math>x (x^3+2 x^2-x-1)=0</math>의 해가 된다 |
| − | + | ||
| − | |||
| − | |||
| − | + | ||
| − | |||
| − | |||
==역사== | ==역사== | ||
| + | * [[수학사 연표]] | ||
| − | |||
| − | + | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
==메모== | ==메모== | ||
| − | + | ||
| − | + | ||
==관련된 항목들== | ==관련된 항목들== | ||
| 132번째 줄: | 80번째 줄: | ||
* [[톨레미의 정리]] | * [[톨레미의 정리]] | ||
| − | |||
| − | + | ==매스매티카 파일 및 계산 리소스== | |
| + | * http://www.wolframalpha.com/input/?i=regular+heptagon+inscribed+in+a+unit+circle | ||
| − | |||
| − | + | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | ==사전 형태의 자료== | |
| − | |||
| − | |||
| − | |||
| − | ==사전 | ||
* [http://ko.wikipedia.org/wiki/%EC%B9%A0%EA%B0%81%ED%98%95 http://ko.wikipedia.org/wiki/칠각형] | * [http://ko.wikipedia.org/wiki/%EC%B9%A0%EA%B0%81%ED%98%95 http://ko.wikipedia.org/wiki/칠각형] | ||
* http://en.wikipedia.org/wiki/Heptagon | * http://en.wikipedia.org/wiki/Heptagon | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | ||
| − | + | ||
==관련논문== | ==관련논문== | ||
| − | * [http://www.ams.org/bull/2005-42-02/S0273-0979-05-01047-5/home.html#References Continued fractions and modular functions] | + | * [http://www.ams.org/bull/2005-42-02/S0273-0979-05-01047-5/home.html#References Continued fractions and modular functions] |
| − | ** W. Duke, | + | ** W. Duke, Bull. Amer. Math. Soc. 42 (2005), 137-162 |
| − | * [http://www.jstor.org/stable/2691048 Golden Fields: A Case for the Heptagon] | + | * [http://www.jstor.org/stable/2691048 Golden Fields: A Case for the Heptagon] |
** Peter Steinbach, Mathematics Magazine Vol. 70, No. 1 (Feb., 1997), pp. 22-31 | ** Peter Steinbach, Mathematics Magazine Vol. 70, No. 1 (Feb., 1997), pp. 22-31 | ||
| − | * [http://dx.doi.org/10.1093/qmath/os-8.1.39 A Note on Spence's Logarithmic Transcendent] | + | * [http://dx.doi.org/10.1093/qmath/os-8.1.39 A Note on Spence's Logarithmic Transcendent] |
| − | ** Watson, G. N., | + | ** Watson, G. N., Quart. J. Math. Oxford Ser. 8, 39-42, 1937 |
| − | * [http://www.jstor.org/stable/3605379 ABCDEFG Is a Regular Heptagon in a Circle of Unit Radius; To Prove That AC+AD-AB=√7] | + | * [http://www.jstor.org/stable/3605379 ABCDEFG Is a Regular Heptagon in a Circle of Unit Radius; To Prove That AC+AD-AB=√7] |
** T. S. Tufton, The Mathematical Gazette Vol. 18, No. 230 (Oct., 1934), pp. 274-275 | ** T. S. Tufton, The Mathematical Gazette Vol. 18, No. 230 (Oct., 1934), pp. 274-275 | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | ==메타데이터== | |
| − | + | ===위키데이터=== | |
| − | * | + | * ID : [https://www.wikidata.org/wiki/Q188866 Q188866] |
| − | + | ===Spacy 패턴 목록=== | |
| − | * [ | + | * [{'LEMMA': 'heptagon'}] |
| − | * [ | + | * [{'LEMMA': '7-gon'}] |
2021년 2월 17일 (수) 04:59 기준 최신판
개요
- 정칠각형에 관련된 여러 가지 수학적 주제의 이해
정칠각형 꼭지점의 평면좌표
- 정칠각형이 단위원에 내접하고 있고, 한 점의 좌표가 \((1,0)\)으로 주어진 경우
- 방정식 \(z^6 + z^5 + z^4 + z^3 + z^2 + z + 1 = 0\)은 다음과 같은 순서로 풀수 있음.
- 양변을 \(z^3\)으로 나누면, \(z^3+z^2+z+1+\frac{1}{z}+\frac{1}{z^2}+\frac{1}{z^3}=0\) 을 얻게됨.
- \(y=z+\frac{1}{z}\) 로 치환하면, 원래의 방정식에서 다음 식을 얻을 수 있음.
\[z^3+z^2+z+1+\frac{1}{z}+\frac{1}{z^2}+\frac{1}{z^3}=(z+\frac{1}{z})^3+(z+\frac{1}{z})^2-2(z+\frac{1}{z})-1=y^3+y^2-2y-1=0\] 3차 방정식의 근의 공식을 이용하여 방정식 \(y^3+y^2-2y-1=0\)을 풀면, 다음을 얻는다 \[y_1= \frac{1}{3} \left(-1+\frac{7^{2/3}}{\sqrt[3]{\frac{1}{2} \left(1+3 i \sqrt{3}\right)}}+\sqrt[3]{\frac{7}{2} \left(1+3 i \sqrt{3}\right)}\right)(=2\cos\frac{2\pi}{7})\] \[y_2=-\frac{1}{3}-\frac{7^{2/3} \left(1+i \sqrt{3}\right)}{3\ 2^{2/3} \sqrt[3]{1+3 i \sqrt{3}}}+\frac{1}{6} i \sqrt[3]{\frac{7}{2} \left(1+3 i \sqrt{3}\right)} \left(\sqrt{3}+i\right)(=2\cos\frac{4\pi}{7})\] \[y_3=-\frac{1}{3}-\frac{1}{6} \left(1+i \sqrt{3}\right) \sqrt[3]{\frac{7}{2} \left(1+3 i \sqrt{3}\right)}+\frac{i 7^{2/3} \left(\sqrt{3}+i\right)}{3\ 2^{2/3} \sqrt[3]{1+3 i \sqrt{3}}}(=2\cos\frac{6\pi}{7})\] 이제 \(z^2-yz+1=0\)로부터 \(z=\frac{y+\sqrt{y^2-4}}{2}\)
정칠각형의 대각선의 길이
- 한 변의 길이가 1인 정칠각형의 대각선의 길이 \(r_i\)는 \(i=0,1,\cdots,5\)에 대하여 다음과 같이 주어짐 \[r_i=\frac{\sin \frac{(i+1)\pi}{7}}{\sin \frac{\pi}{7}}\] * 이 때 \(r_0=1\), \(r_5=1\)
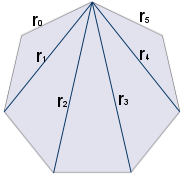
- 제2종 체비셰프 다항식
- 대각선이 만족시키는 다양한 항등식\[r_hr_k=r_{h-k}+r_{h-k+2}+\cdots+r_{h+k}, 0\leq k\leq h\leq 2\]\[r_i^2=1+r_{i-1}r_{i+1}, 1\leq i \leq 4\]\[r_0r_0=r_0\]\[r_1r_0=r_1\]\[r_1r_1=r_0+r_2\]\[r_2r_0=r_2\]\[r_2r_1=r_1+r_3\]\[r_2r_2=r_0+r_2+r_4\]
- \(r_1\)은 \(x^3-x^2-2x+1=0\) 의 해이다 http://www.wolframalpha.com/input/?i=+%28sin%282pi%2F7%29%2Fsin%28pi%2F7%29%29
(증명)
\(r_1r_1=r_0+r_2\)
\(r_2r_1=r_1+r_3=r_1+r_2\)■
- \(r_2\)은 \(x^3-2x^2-x+1=0\) 의 해이다 http://www.wolframalpha.com/input/?i=+%28sin%283pi%2F7%29%2Fsin%28pi%2F7%29%29
(증명)
\(r_1r_1=r_0+r_2\)
\(r_2r_2=r_0+r_2+r_4=r_0+r_2+r_1\) ■
다이로그 항등식
- 다이로그 항등식 (dilogarithm identities)\[\alpha=\frac{\sec\frac{2\pi}{7}}{2}=0.80194\cdots\]\[\beta=\frac{\sec\frac{\pi}{7}}{2}=0.554958\cdots\]\[\gamma=2\cos\frac{3\pi}{7}=0.445042\cdots\]\[7L(\alpha^2)-7L(\alpha)+L(1)=0\]\[7L(\beta^2)+14L(\beta)-10L(1)=0\]\[7L(\gamma^2)+14L(\gamma)-8L(1)=0\]\[\sum_{i=1}^{5}L\left(\frac{\sin^2\frac{\pi}{7}}{\sin^2\frac{(i+1)\pi}{7}}\right)=\frac{5\pi^2}{14}\]
- 방정식 \(x^3+2x^2-x-1=0\) 의 해 \(\alpha, -\beta, -\gamma^{-1}\)
- 방정식 \(x^3+x^2-2x-1=0\)의 해 \(a,b,c\)
\(a=2\cos\frac{2\pi}{7}=\alpha^{-1}=1.24698\cdots\), \(b=2\cos\frac{4\pi}{7}=-\gamma=-0.445042\cdots\),\(c=2\cos\frac{6\pi}{7}=-\beta^{-1}=-1.80194\cdots\)
- k=3인 경우의 앤드류스-고든 항등식(Andrews-Gordon identity)\[1-x_{1}=x_{1}^{4}x_{2}^{2}\]\[1-x_{2}=x_{1}^{2}x_{2}^{2}\]를 풀면,\[x_{2}=1-\frac{1-x_{1}}{x_{1}^{2}}=\frac{x_{1}^{2}+x_{1}-1}{x_{1}^{2}}\]\[1-x_{1}=(x_{1}^{2}+x_{1}-1)^{2}\] 따라서 \(x_1\)은 \(x (x^3+2 x^2-x-1)=0\)의 해가 된다
역사
메모
관련된 항목들
매스매티카 파일 및 계산 리소스
사전 형태의 자료
관련논문
- Continued fractions and modular functions
- W. Duke, Bull. Amer. Math. Soc. 42 (2005), 137-162
- Golden Fields: A Case for the Heptagon
- Peter Steinbach, Mathematics Magazine Vol. 70, No. 1 (Feb., 1997), pp. 22-31
- A Note on Spence's Logarithmic Transcendent
- Watson, G. N., Quart. J. Math. Oxford Ser. 8, 39-42, 1937
- ABCDEFG Is a Regular Heptagon in a Circle of Unit Radius; To Prove That AC+AD-AB=√7
- T. S. Tufton, The Mathematical Gazette Vol. 18, No. 230 (Oct., 1934), pp. 274-275
메타데이터
위키데이터
- ID : Q188866
Spacy 패턴 목록
- [{'LEMMA': 'heptagon'}]
- [{'LEMMA': '7-gon'}]