클라인의 4차곡선

수학노트
둘러보기로 가기 검색하러 가기

개요

  • 종수(genus)가 3인 복소대수곡선
    • \(\mathbb CP^2\) 에서 \(x^3y+y^3z+z^3x=0\) 로 주어진 (복소) 대수곡선
    • 푸앵카레 상반평면을 universal covering으로 갖는 쌍곡기하학의 곡면\[\mathbb H^2/\Gamma(7)\]\[\Gamma(7)=\left\{\begin{bmatrix} a&b \\ c&d \end{bmatrix} \in SL(2,\mathbb Z) : \begin{bmatrix} a&b \\ c&d \end{bmatrix} \equiv \begin{bmatrix} 1&0\\ 0&1 \end{bmatrix} \pmod 7\right\}\]
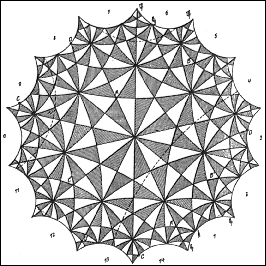
  • 쌍곡기하학 세계의 플라톤 다면체(Platonic solid), 즉 정다면체
    • 정칠각형으로 24조각으로 만들어진 정이십사면체
    • 자기동형군, 즉 대칭군은 \(\operatorname{PSL}(2,\mathbb{F}_ 7)\)와 동형임.
    • 168가지의 대칭을 가짐


주기 행렬

\[ \frac{1}{2} \left( \begin{array}{ccc} \rho & 1 & 1 \\ 1 & \rho & 1 \\ 1 & 1 & \rho \\ \end{array} \right) \] 여기서 \(\rho=\frac{-1+\sqrt{-7}}{2}\).


자기동형군

  • \(x^3y+y^3z+z^3x=0\)
  • order 3 x-> y-> z-> x
  • order 7 x->ax, y->by, z->cz for some a,b,c we want \(a^3b=b^3c=c^3a\) solution \[ a=\zeta^4, b=\zeta^2,c=\zeta^1\] where \(\zeta^7=1\)


PSL(2,7)

  • PSL(2,7) (isomorphic PSL(3,2)) has order 168
  • GL(2,7) has order \((7^2-1)(7^2-7)\)
  • SL(2,7) has order \((7^2-1)7=6\times 7\times 8\)
  • PSL(2,7) has order \(6\times 7\times 8/2\)
  • 크기가 가장 작은 후르비츠 군이다 \(a^2=b^3=c^7=abc=1\). 여기서 \(a=S, b=ST, c=T\) 로 두면 된다 (S,T는 모듈라 군(modular group) 의 원소)

\[S=\begin{pmatrix} 0 & -1 \\ 1 & 0 \end{pmatrix},T=\begin{pmatrix} 1 & 1 \\ 0 & 1 \end{pmatrix} \]

  • PSL(2,7)은 3차원 표현을 가지므로 \( \mathbb{C}[x,y,z]\)에 작용한다.
  • any polynomial invariant under PSL(2,7) also invariant under subgroup of order 21.
  • (generated by order 3 transformation \(x\to y\to z\to x\) and order 7 transformation \(x\to ax, y\to by, z\to cz\) where \(a=\zeta^4, b=\zeta^2,c=\zeta^1\) where \(\zeta^7=1\))
  • Not many invariant elements of degree 4.
  • Only monomials of degree 4 invariant under elements of order 7 are \(x^3y,y^3z,z^3x\).
  • If in addition we require invariance under \(x\to y\to z\to x\), only possibility is \(\text{constant} \times (x^3y+y^3z+z^3x)\).
  • If any Hurwitz group acts on a curve, then PSL(2,7) acts on \(x^3y+y^3z+z^3x=0\)


(2,3,7) 삼각형

  • 삼각형의 세 각이 각각\[\frac{\pi}{7},\frac{\pi}{3},\frac{\pi}{2}\] 로 주어지며, 이 세각의 크기를 모두 더하면,\[\frac{\pi}{7}+\frac{\pi}{3}+\frac{\pi}{2}=\frac{41\pi}{42}\] 가 되어, 180도보다 작게 된다.
  • 쌍곡기하학에서의 곡률은 음수이기 때문에 나타나는 현상이다.
  • 쌍곡기하학 항목 참조



전개도

3063024-klein.gif



세타함수

  • 세타함수\[\theta_{7,1}=\sum_{k\in Z}(-1)^{k}q^{\frac{(14k+1)^2}{56}}=q^{1/56}(q^3;q^7)_\infty (q^4; q^7)_\infty(q^7;q^7)_\infty\]\[\theta_{7,3}=\sum_{k\in Z}(-1)^{k}q^{\frac{(14k+3)^2}{56}}=q^{9/56}(q^2;q^7)_\infty (q^5; q^7)_\infty(q^7;q^7)_\infty\]\[\theta_{7,5}=\sum_{k\in Z}(-1)^{k}q^{\frac{(14k+5)^2}{56}}=q^{25/56}(q;q^7)_\infty (q^6; q^7)_\infty(q^7;q^7)_\infty\]
  • 세타함수는 클라인 곡선을 매개화한다\[x=\theta_{7,1},y=-\theta_{7,5},z=\theta_{7,3}\]

\[x^3y+y^3z+z^3x=0\] \[xyz=-\eta(\tau)\eta(7\tau)^2\]



조각

3063024-DSCN4142.JPG

메모


매스매티카 파일 및 계산 리소스


관련된 항목들



사전형태의 자료


관련도서



관련논문

블로그

메타데이터

위키데이터

Spacy 패턴 목록

  • [{'LOWER': 'klein'}, {'LEMMA': 'quartic'}]
원본 주소 "https://wiki.mathnt.net/index.php?title=클라인의_4차곡선&oldid=52503"