"정다각형의 대각선의 길이"의 두 판 사이의 차이
Pythagoras0 (토론 | 기여) |
|||
| (사용자 2명의 중간 판 29개는 보이지 않습니다) | |||
| 1번째 줄: | 1번째 줄: | ||
| − | < | + | ==개요== |
| + | * 한 변의 길이가 1인 정n각형의 대각선의 길이 | ||
| + | * 편의상 <math>n=\ell+2</math>로 두자. 대각선의 길이 <math>r_i</math>는 다음과 같이 주어진다 | ||
| + | :<math>r_i=\frac{\sin \left(\frac{\pi (i+1)}{\ell+2}\right)}{\sin \left(\frac{\pi }{\ell+2}\right)},\quad i=0,1,\cdots,\ell</math> | ||
| + | * <math>r_0=1</math>, <math>r_{\ell}=1</math> 임을 확인할 수 있다 | ||
| + | * [[톨레미의 정리]] | ||
| + | * 그림은 [[정칠각형]] 의 경우 | ||
| + | [[파일:6782509-heptagon.png]] | ||
| − | |||
| − | + | ==대각선이 만족시키는 항등식 1== | |
| + | * 다음을 만족한다 | ||
| + | :<math>r_h\times r_k=r_{h-k}+r_{h-k+2}+\cdots+r_{h+k} \label{pd}</math> | ||
| + | 여기서 <math>0\leq k\leq h<n/2</math>이고, 우변은 k+1개항의 합. | ||
| + | * 다음과 같이 쓸 수 있다 | ||
| + | :<math> | ||
| + | r_{h}\times r_{k}= r_{|h-k|}+ r_{|h-k|+2}+ \cdots + r_{\operatorname{min}(2\ell-(h+k),h+k)} | ||
| + | </math> | ||
| + | 여기서 <math>0\leq h,k \leq \ell</math> | ||
| + | * 고등수학의 등각장론에서 fusion rule 로 등장한다 | ||
| − | |||
| − | |||
| − | + | ===증명=== | |
| − | |||
| − | + | 다음과 같은 [[삼각함수의 덧셈과 곱셈 공식]] 을 이용하자. | |
| + | :<math>\sin{x} \sin{y} = -{\cos(x + y) - \cos(x - y) \over 2}</math> | ||
| + | 이를 이용하여, 다음을 얻을 수 있다 | ||
| + | :<math>\sin \frac{(h+1)\pi}{n}\sin \frac{(k+1)\pi}{n}=\sum_{j=0}^{k}\sin \frac{(h-k+2j+1)\pi}{n}\sin \frac{\pi}{n}</math> | ||
| + | 따라서 | ||
| + | :<math>r_h\times r_k=r_{h-k}+r_{h-k+2}+\cdots+r_{h+k}</math> ■ | ||
| + | |||
| − | + | ==대각선이 만족시키는 항등식 2== | |
| + | * <math>r_i</math>는 다음의 점화식을 만족한다 | ||
| + | :<math>r_i^2=1+r_{i-1}r_{i+1}, 1\leq i \leq \ell-1</math> | ||
| + | * \ref{pd}을 이용하여 증명할 수 있다 | ||
| + | * 이는 제2종 [[체비셰프 다항식]]이 만족시키는 항등식 <math>U_n(x)^2=1+U_{n-1}(x)U_{n+1}(x)</math>과 같다 | ||
| − | |||
| − | |||
| − | + | ==양자미적분학== | |
| − | [[ | + | * [[q-초기하급수(q-hypergeometric series)와 양자미적분학(q-calculus)]] 에서 실수 n 의 q-analogue 로:<math>[n]_q =\frac{q^{n}-q^{-n}}{q-q^{-1}} </math> 와 같은 표현을 사용하기도 함 |
| + | * <math>q=e^{i\theta}</math> 로 두면,:<math>[n]_q =\frac{e^{in\theta}-e^{-in\theta}}{e^{i\theta}-e^{-i\theta}} =\frac{\sin n\theta}{\sin \theta}</math> 를 얻는다 | ||
| + | * 정다각형의 대각선의 길이:<math>r_i=\frac{\sin \left(\frac{\pi (i+1)}{n}\right)}{\sin \left(\frac{\pi }{n}\right)}</math> 와 유사한 표현을 얻는다 | ||
| + | * 이는 [[바일 차원 공식(Weyl dimension formula)]]을 이용하여 quantum dimension을 정의할 때 등장하는 표현이다 | ||
| − | |||
| − | + | ==예== | |
| + | |||
| − | + | ===정사각형의 대각선=== | |
| − | + | * [[루트2는 무리수이다]] | |
| − | + | ||
| − | + | '''정사각형의 대각선의 길이''' | |
| − | + | 한변의 길이가 1인 정사각형의 대각선의 길이는 [[피타고라스의 정리]]를 이용하여 루트 2가 됨을 보일수 있다. "[[루트2는 무리수이다|루트2는 무리수"]] 라는 이야기는 중고교수학에서 배우는 가장 멋진 사실의 하나라 할 수 있다. | |
| − | + | ||
| − | + | ||
| − | + | ===정오각형의 대각선=== | |
| + | ====방법1==== | ||
| − | + | [[정오각형]]의 한 변의 길이와 대각선의 길이의 비율이 [[황금비]]가 된다는 것은 잘 알려진 사실이다. | |
| + | :<math>{b \over a}={{(1+\sqrt{5})}\over 2}</math> | ||
| − | + | [[파일:3002548-pentagon(1).png]] | |
| − | + | 정오각형의 한 변의 길이가 1인 경우, 즉 <math>a=1</math>인 경우에 b는 황금비가 된다. | |
| − | + | ;증명 | |
| − | + | 삼각형 ABD에서 선분 AC는 각 A의 이등분선이다. (각 DAC와 각 CAB가 같은 길이를 갖는 두 현 DC와 BC의 원주각이기 때문) | |
| − | + | AC와 BD의 교점을 E라 하자. | |
| − | + | 각의 이등분선의 성질에 의해, | |
| − | < | + | AB : AD = BE : DE 즉 <math>a : b = b-a : a</math> 가 성립한다. |
| − | + | :<math>b^2 - ab - a^2 = 0</math> | |
| + | :<math>(\frac{b}{a})^2- \frac{b}{a} - 1 =0</math> | ||
| + | ■ | ||
| − | |||
| − | + | ====방법 2==== | |
| − | + | 정오각형의 대각선의 길이를 구하는 또 다른 방법의 하나는 평면기하의 [[톨레미의 정리]]를 이용하는 것이다. 톨레미의 정리는 다음과 같다 : | |
| − | + | 사각형이 원에 내접할때, 두 대각선의 길이의 곱은 서로 마주보고 있는 두 변의 쌍의 길이의 곱의 합과 같다. | |
| − | + | 즉, 아래그림에서 <math>\overline{AC}\cdot \overline{BD}=\overline{AB}\cdot \overline{CD}+\overline{BC}\cdot \overline{AD}</math> 이 성립한다. | |
| − | |||
| − | + | [[파일:3002548-pentagon(1).png]] | |
| − | |||
| − | + | 톨레미의 정리를 적용해 보면, 사각형 ABCD가 원에 내접하고 있으므로, 두 대각선 AC와 BD의 길이의 곱으로부터 <math>\overline{AC}\cdot \overline{BD}=b^2</math>을 얻고, <math>\overline{AB}\cdot \overline{CD}+\overline{BC}\cdot \overline{AD}=a^2+ab</math>를 얻을 수 있다. | |
| − | + | 이로부터 <math>b^2 - ab - a^2 = 0</math>를 얻을 수도 있다. | |
| − | + | ===정육각형의 대각선=== | |
| − | + | ||
| − | + | '''정육각형의 대각선의 길이''' | |
| − | |||
| − | + | 각 변의 길이가 1인 정육각형의 대각선의 길이는 <math>\sqrt{3}</math>과 2가 되는데, 이는 정삼각형의 변의 길이를 구할 수 있다면 어렵지 않게 구할 수 있다. | |
| − | + | ||
| − | + | ||
| − | + | ===정칠각형의 대각선=== | |
| − | + | * [[정칠각형]] | |
| − | * [ | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | '''정칠각형의 대각선의 길이''' | |
| − | + | [[정칠각형|정칠]][[정칠각형|각형]]의 대각선의 길이도 마찬가지로 톨레미의 정리를 여러번 적용하면 구할 수 있다. | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | [[파일:6782509-heptagon.png]] | |
| − | < | + | 한변의 길이 <math>r_0=1</math> 라 두고, 톨레미의 정리를 적용하면, |
| − | + | <math>r_1^2=1+r_2</math> | |
| − | + | <math>r_2r_1=r_1+r_2</math> | |
| − | < | + | <math>r_2^2=r_2r_1+1</math> |
| − | + | 와 같은 관계를 얻을 수 있다. | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | ||
| − | + | 이를 이용하면, 다음과 같은 사실들을 알 수 있다. | |
| − | < | + | <math>r_1</math>은 <math>x^3-x^2-2x+1=0</math> 의 해이고, <math>r_2</math>은 <math>x^3-2x^2-x+1=0</math> 의 해이다. |
| − | + | (증명) | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | <math>r_2=r_1^2-1</math> 이므로, <math>r_2r_1=r_1+r_2</math>로부터 <math>r_1(r_1^2-1)=r_1+r_1^2-1</math>. | |
| − | + | <math>r_2^2=r_2r_1+1=r_1+r_2+1</math> 이므로, <math>r_1=r_2^2-r_2-1</math>. 이제 <math>r_2r_1=r_1+r_2</math>로부터, <math>r_2(r_2^2-r_2-1)=r_2^2-r_2-1+r_2</math>. ■ | |
| − | + | 이제 3차 방정식을 풀면 된다. | |
| + | |||
| − | * http://www. | + | ==메모== |
| − | * http:// | + | * [http://bomber0.byus.net/index.php/2010/12/22/1862 정다각형의 대각선의 길이], 피타고라스의 창 |
| − | * http:// | + | * [http://www.math.rutgers.edu/%7Eerowland/polygons.html http://www.math.rutgers.edu/~erowland/polygons.html] |
| + | * http://twistedone151.wordpress.com/2010/10/25/monday-math-140/ | ||
| + | * http://twistedone151.wordpress.com/2010/11/01/monday-math-141/ | ||
| + | * * http://planetmath.org/encyclopedia/ExampleOfTelescopingSum.html | ||
| − | + | ||
| − | + | ||
| + | ==관련된 항목들== | ||
| + | * [[사인곱]] | ||
| + | |||
| − | |||
| − | + | ==관련논문== | |
| − | * | + | * A. Fontaine and S. Hurley, [http://forumgeom.fau.edu/FG2006volume6/FG200610.pdf Proof by picture: Products and reciprocals of diagonal length ratios in the regular polygon], Forum Geom., 6 (2006) 97–101. |
| − | + | * Lang, Wolfdieter. 2012. “The Field Q(2cos(pi/n)), Its Galois Group and Length Ratios in the Regular N-gon.” arXiv:1210.1018 (October 3). http://arxiv.org/abs/1210.1018. | |
| − | + | * [http://forumgeom.fau.edu/FG2006volume6/FG200630.pdf Formulas among diagonals in the regular polygon and the Catalan numbers] | |
| − | + | ||
| − | * | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | * | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | ==매스매티카 파일 및 계산 리소스== | |
| − | * | + | * https://docs.google.com/open?id=0B8XXo8Tve1cxWDN2enlhYXdTbTZ6UDB6VWtIOEFVUQ |
| − | |||
| − | |||
| − | |||
| − | |||
2020년 11월 13일 (금) 07:14 기준 최신판
개요
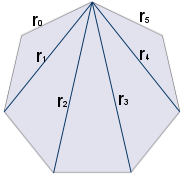
- 한 변의 길이가 1인 정n각형의 대각선의 길이
- 편의상 \(n=\ell+2\)로 두자. 대각선의 길이 \(r_i\)는 다음과 같이 주어진다
\[r_i=\frac{\sin \left(\frac{\pi (i+1)}{\ell+2}\right)}{\sin \left(\frac{\pi }{\ell+2}\right)},\quad i=0,1,\cdots,\ell\]
대각선이 만족시키는 항등식 1
- 다음을 만족한다
\[r_h\times r_k=r_{h-k}+r_{h-k+2}+\cdots+r_{h+k} \label{pd}\] 여기서 \(0\leq k\leq h<n/2\)이고, 우변은 k+1개항의 합.
- 다음과 같이 쓸 수 있다
\[ r_{h}\times r_{k}= r_{|h-k|}+ r_{|h-k|+2}+ \cdots + r_{\operatorname{min}(2\ell-(h+k),h+k)} \] 여기서 \(0\leq h,k \leq \ell\)
- 고등수학의 등각장론에서 fusion rule 로 등장한다
증명
다음과 같은 삼각함수의 덧셈과 곱셈 공식 을 이용하자. \[\sin{x} \sin{y} = -{\cos(x + y) - \cos(x - y) \over 2}\] 이를 이용하여, 다음을 얻을 수 있다 \[\sin \frac{(h+1)\pi}{n}\sin \frac{(k+1)\pi}{n}=\sum_{j=0}^{k}\sin \frac{(h-k+2j+1)\pi}{n}\sin \frac{\pi}{n}\] 따라서 \[r_h\times r_k=r_{h-k}+r_{h-k+2}+\cdots+r_{h+k}\] ■
대각선이 만족시키는 항등식 2
- \(r_i\)는 다음의 점화식을 만족한다
\[r_i^2=1+r_{i-1}r_{i+1}, 1\leq i \leq \ell-1\]
- \ref{pd}을 이용하여 증명할 수 있다
- 이는 제2종 체비셰프 다항식이 만족시키는 항등식 \(U_n(x)^2=1+U_{n-1}(x)U_{n+1}(x)\)과 같다
양자미적분학
- q-초기하급수(q-hypergeometric series)와 양자미적분학(q-calculus) 에서 실수 n 의 q-analogue 로\[[n]_q =\frac{q^{n}-q^{-n}}{q-q^{-1}} \] 와 같은 표현을 사용하기도 함
- \(q=e^{i\theta}\) 로 두면,\[[n]_q =\frac{e^{in\theta}-e^{-in\theta}}{e^{i\theta}-e^{-i\theta}} =\frac{\sin n\theta}{\sin \theta}\] 를 얻는다
- 정다각형의 대각선의 길이\[r_i=\frac{\sin \left(\frac{\pi (i+1)}{n}\right)}{\sin \left(\frac{\pi }{n}\right)}\] 와 유사한 표현을 얻는다
- 이는 바일 차원 공식(Weyl dimension formula)을 이용하여 quantum dimension을 정의할 때 등장하는 표현이다
예
정사각형의 대각선
정사각형의 대각선의 길이
한변의 길이가 1인 정사각형의 대각선의 길이는 피타고라스의 정리를 이용하여 루트 2가 됨을 보일수 있다. "루트2는 무리수" 라는 이야기는 중고교수학에서 배우는 가장 멋진 사실의 하나라 할 수 있다.
정오각형의 대각선
방법1
정오각형의 한 변의 길이와 대각선의 길이의 비율이 황금비가 된다는 것은 잘 알려진 사실이다. \[{b \over a}={{(1+\sqrt{5})}\over 2}\]
정오각형의 한 변의 길이가 1인 경우, 즉 \(a=1\)인 경우에 b는 황금비가 된다.
- 증명
삼각형 ABD에서 선분 AC는 각 A의 이등분선이다. (각 DAC와 각 CAB가 같은 길이를 갖는 두 현 DC와 BC의 원주각이기 때문)
AC와 BD의 교점을 E라 하자.
각의 이등분선의 성질에 의해,
AB : AD = BE : DE 즉 \(a : b = b-a : a\) 가 성립한다.
\[b^2 - ab - a^2 = 0\] \[(\frac{b}{a})^2- \frac{b}{a} - 1 =0\] ■
방법 2
정오각형의 대각선의 길이를 구하는 또 다른 방법의 하나는 평면기하의 톨레미의 정리를 이용하는 것이다. 톨레미의 정리는 다음과 같다 :
사각형이 원에 내접할때, 두 대각선의 길이의 곱은 서로 마주보고 있는 두 변의 쌍의 길이의 곱의 합과 같다.
즉, 아래그림에서 \(\overline{AC}\cdot \overline{BD}=\overline{AB}\cdot \overline{CD}+\overline{BC}\cdot \overline{AD}\) 이 성립한다.
톨레미의 정리를 적용해 보면, 사각형 ABCD가 원에 내접하고 있으므로, 두 대각선 AC와 BD의 길이의 곱으로부터 \(\overline{AC}\cdot \overline{BD}=b^2\)을 얻고, \(\overline{AB}\cdot \overline{CD}+\overline{BC}\cdot \overline{AD}=a^2+ab\)를 얻을 수 있다.
이로부터 \(b^2 - ab - a^2 = 0\)를 얻을 수도 있다.
정육각형의 대각선
정육각형의 대각선의 길이
각 변의 길이가 1인 정육각형의 대각선의 길이는 \(\sqrt{3}\)과 2가 되는데, 이는 정삼각형의 변의 길이를 구할 수 있다면 어렵지 않게 구할 수 있다.
정칠각형의 대각선
정칠각형의 대각선의 길이
정칠각형의 대각선의 길이도 마찬가지로 톨레미의 정리를 여러번 적용하면 구할 수 있다.
한변의 길이 \(r_0=1\) 라 두고, 톨레미의 정리를 적용하면,
\(r_1^2=1+r_2\)
\(r_2r_1=r_1+r_2\)
\(r_2^2=r_2r_1+1\)
와 같은 관계를 얻을 수 있다.
이를 이용하면, 다음과 같은 사실들을 알 수 있다.
\(r_1\)은 \(x^3-x^2-2x+1=0\) 의 해이고, \(r_2\)은 \(x^3-2x^2-x+1=0\) 의 해이다.
(증명)
\(r_2=r_1^2-1\) 이므로, \(r_2r_1=r_1+r_2\)로부터 \(r_1(r_1^2-1)=r_1+r_1^2-1\).
\(r_2^2=r_2r_1+1=r_1+r_2+1\) 이므로, \(r_1=r_2^2-r_2-1\). 이제 \(r_2r_1=r_1+r_2\)로부터, \(r_2(r_2^2-r_2-1)=r_2^2-r_2-1+r_2\). ■
이제 3차 방정식을 풀면 된다.
메모
- 정다각형의 대각선의 길이, 피타고라스의 창
- http://www.math.rutgers.edu/~erowland/polygons.html
- http://twistedone151.wordpress.com/2010/10/25/monday-math-140/
- http://twistedone151.wordpress.com/2010/11/01/monday-math-141/
- * http://planetmath.org/encyclopedia/ExampleOfTelescopingSum.html
관련된 항목들
관련논문
- A. Fontaine and S. Hurley, Proof by picture: Products and reciprocals of diagonal length ratios in the regular polygon, Forum Geom., 6 (2006) 97–101.
- Lang, Wolfdieter. 2012. “The Field Q(2cos(pi/n)), Its Galois Group and Length Ratios in the Regular N-gon.” arXiv:1210.1018 (October 3). http://arxiv.org/abs/1210.1018.
- Formulas among diagonals in the regular polygon and the Catalan numbers