"드 무아브르의 정리, 복소수와 정다각형"의 두 판 사이의 차이
둘러보기로 가기
검색하러 가기
Pythagoras0 (토론 | 기여) |
|||
| (사용자 2명의 중간 판 17개는 보이지 않습니다) | |||
| 1번째 줄: | 1번째 줄: | ||
| − | + | ==개요== | |
| + | |||
| + | (정리) 드 무아브르 | ||
<math>(\cos \theta + i \sin \theta)^n=\cos n\theta + i \sin n\theta</math> | <math>(\cos \theta + i \sin \theta)^n=\cos n\theta + i \sin n\theta</math> | ||
| − | + | 여기서 <math>\theta</math> 는 임의의 실수, <math>n</math> 은 임의의 정수 | |
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| + | ==증명== | ||
| + | |||
| + | * 수학적 귀납법 | ||
| − | + | ||
| − | + | ||
| − | |||
| − | |||
| − | |||
| − | + | ==오일러의 정리를 통한 증명== | |
| − | + | * [[오일러의 공식]] | |
| + | * 복소지수함수 <math>e^{i\theta}=\cos \theta+ i\sin \theta</math> 의 성질에서 자연스럽게 유도 | ||
| − | + | <math>(\cos \theta+ i\sin \theta)^n=(e^{i\theta})^n=e^{in\theta}= \cos n\theta+ i\sin n\theta</math> | |
| − | + | ||
| − | + | ||
| − | + | ||
| − | |||
| − | + | ||
| − | + | ==정다각형과의 관계== | |
| − | < | + | * <math>z^n=1</math> 를 만족시키는 복소수 방정식을 풀면, n개의 해는 복소평면에서 정n각형의 꼭지점이 된다. 방정식을 풀기 위해, <math>z=\cos \theta + i \sin \theta</math> 로 두고 드 무아브르 정리를 적용하자.:<math>(\cos \theta + i \sin \theta)^n=\cos n\theta + i \sin n\theta=1</math>:<math>\theta=\frac{2k\pi}{n}, k=0,1,\cdots,n-1</math> |
| + | * <math>z^3=1</math> 의 해는, <math>1,\frac{-1+\sqrt{-3}}{2}, \frac{-1-\sqrt{-3}}{2}</math> 세 개가 있다. 이를 복소평면에 점으로 나타내면, 다음과 같이 정삼각형의 꼭지점을 이룬다.[[파일:3002568-img602.gif]] | ||
| − | + | ||
| − | + | ||
| − | + | ==많이 나오는 질문== | |
| − | * 네이버 지식인 | + | * 네이버 지식인 |
** [http://kin.search.naver.com/search.naver?where=kin_qna&query=%EB%93%9C%EB%AC%B4%EC%95%84%EB%B8%8C%EB%A5%B4 http://kin.search.naver.com/search.naver?where=kin_qna&query=드무아브르] | ** [http://kin.search.naver.com/search.naver?where=kin_qna&query=%EB%93%9C%EB%AC%B4%EC%95%84%EB%B8%8C%EB%A5%B4 http://kin.search.naver.com/search.naver?where=kin_qna&query=드무아브르] | ||
| − | + | ||
| − | + | ==관련된 고교수학 또는 대학수학== | |
* 삼각함수 | * 삼각함수 | ||
* [[복소수]] | * [[복소수]] | ||
* [[추상대수학]] | * [[추상대수학]] | ||
| − | |||
* [[복소함수론]] | * [[복소함수론]] | ||
| − | + | ||
| + | |||
| + | |||
| − | + | ==관련된 항목들== | |
* [[정다각형의 작도]] | * [[정다각형의 작도]] | ||
* [[정오각형]] | * [[정오각형]] | ||
* [[가우스와 정17각형의 작도]] | * [[가우스와 정17각형의 작도]] | ||
| − | * [[ | + | * [[수학사 연표]] |
* [[오일러의 공식 e^{iπ}+1=0|오일러의 공식]] | * [[오일러의 공식 e^{iπ}+1=0|오일러의 공식]] | ||
| + | * [[정규분포와 그 확률밀도함수|정규분포와 중심극한정리]] | ||
| − | + | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | ||
| − | + | ==사전형태의 자료== | |
* [http://ko.wikipedia.org/wiki/%EB%B3%B5%EC%86%8C%EC%88%98 http://ko.wikipedia.org/wiki/복소수] | * [http://ko.wikipedia.org/wiki/%EB%B3%B5%EC%86%8C%EC%88%98 http://ko.wikipedia.org/wiki/복소수] | ||
| − | * http://en.wikipedia.org/wiki/ | + | * http://en.wikipedia.org/wiki/De_Moivre |
| − | * http:/ | + | * [http://en.wikipedia.org/wiki/De_Moivre%27s_formula http://en.wikipedia.org/wiki/De_Moivre's_formula] |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | ||
| − | + | ||
| − | |||
| − | |||
| − | + | ==관련기사== | |
| − | + | * 네이버 뉴스 검색 (키워드 수정) | |
| + | ** [http://news.search.naver.com/search.naver?where=news&x=0&y=0&sm=tab_hty&query=%EB%93%9C%EB%AC%B4%EC%95%84%EB%B8%8C%EB%A5%B4 http://news.search.naver.com/search.naver?where=news&x=0&y=0&sm=tab_hty&query=드무아브르] | ||
| + | ** http://news.search.naver.com/search.naver?where=news&x=0&y=0&sm=tab_hty&query= | ||
| + | [[분류:리만곡면론]] | ||
| + | [[분류:복소함수론]] | ||
| − | * | + | ==메타데이터== |
| + | ===위키데이터=== | ||
| + | * ID : [https://www.wikidata.org/wiki/Q200397 Q200397] | ||
| + | ===Spacy 패턴 목록=== | ||
| + | * [{'LOWER': 'abraham'}, {'LOWER': 'de'}, {'LEMMA': 'Moivre'}] | ||
| + | * [{'LEMMA': 'Feo'}] | ||
| + | * [{'LOWER': 'abraham'}, {'LEMMA': 'Demoivre'}] | ||
2021년 2월 17일 (수) 05:03 기준 최신판
개요
(정리) 드 무아브르
\((\cos \theta + i \sin \theta)^n=\cos n\theta + i \sin n\theta\)
여기서 \(\theta\) 는 임의의 실수, \(n\) 은 임의의 정수
증명
- 수학적 귀납법
오일러의 정리를 통한 증명
- 오일러의 공식
- 복소지수함수 \(e^{i\theta}=\cos \theta+ i\sin \theta\) 의 성질에서 자연스럽게 유도
\((\cos \theta+ i\sin \theta)^n=(e^{i\theta})^n=e^{in\theta}= \cos n\theta+ i\sin n\theta\)
정다각형과의 관계
- \(z^n=1\) 를 만족시키는 복소수 방정식을 풀면, n개의 해는 복소평면에서 정n각형의 꼭지점이 된다. 방정식을 풀기 위해, \(z=\cos \theta + i \sin \theta\) 로 두고 드 무아브르 정리를 적용하자.\[(\cos \theta + i \sin \theta)^n=\cos n\theta + i \sin n\theta=1\]\[\theta=\frac{2k\pi}{n}, k=0,1,\cdots,n-1\]
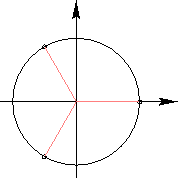
- \(z^3=1\) 의 해는, \(1,\frac{-1+\sqrt{-3}}{2}, \frac{-1-\sqrt{-3}}{2}\) 세 개가 있다. 이를 복소평면에 점으로 나타내면, 다음과 같이 정삼각형의 꼭지점을 이룬다.
많이 나오는 질문
관련된 고교수학 또는 대학수학
관련된 항목들
- 정다각형의 작도
- 정오각형
- 가우스와 정17각형의 작도
- 수학사 연표
- [[오일러의 공식 e^{iπ}+1=0|오일러의 공식]]
- 정규분포와 중심극한정리
사전형태의 자료
- http://ko.wikipedia.org/wiki/복소수
- http://en.wikipedia.org/wiki/De_Moivre
- http://en.wikipedia.org/wiki/De_Moivre's_formula
관련기사
- 네이버 뉴스 검색 (키워드 수정)
메타데이터
위키데이터
- ID : Q200397
Spacy 패턴 목록
- [{'LOWER': 'abraham'}, {'LOWER': 'de'}, {'LEMMA': 'Moivre'}]
- [{'LEMMA': 'Feo'}]
- [{'LOWER': 'abraham'}, {'LEMMA': 'Demoivre'}]