"원주율(파이,π)"의 두 판 사이의 차이
둘러보기로 가기
검색하러 가기
Pythagoras0 (토론 | 기여) |
|||
| (사용자 3명의 중간 판 53개는 보이지 않습니다) | |||
| 1번째 줄: | 1번째 줄: | ||
| − | + | ==개요== | |
| − | * | + | * 원주율(파이,π)는 원의 둘레와 지름의 비율 |
| − | + | * 모든 원은 서로 닮음이므로, 이 비율은 상수이다 | |
| − | + | ||
| − | [ | + | [[파일:2519130-circle_diagram1.jpg]] |
| − | * 3. | + | * <math>\pi=3.141592653589793238462643383279502884197169399375\cdots</math> |
| + | * 수학의 수많은 곳에서 등장한다 | ||
| − | + | ==표기법의 역사== | |
| + | * http://collation.folger.edu/2015/03/pi-day-represented/ | ||
| + | * The first time we see the Greek letter π used in connection with circles is in Oughtred’s 1647 Key of the Mathematics. Here, Oughtred used π to represent the periphery (or circumference) of a circle and ∂ to represent the diameter in the ratio. | ||
| + | * It wasn’t until the beginning of the 18th century that π started to be used in its current way, thanks to to William Jones’s A New Introduction to the Mathematics | ||
| − | |||
| − | + | ==원주율의 계산== | |
| + | * http://numbers.computation.free.fr/Constants/Pi/piCompute.html | ||
| + | * http://en.wikipedia.org/wiki/Chronology_of_computation_of_%CF%80 | ||
| − | |||
| − | + | ===아르키메데스의 부등식=== | |
| − | + | * <math>223/71 < \pi < 22/7</math> | |
| − | + | ||
| − | + | ||
| − | |||
| − | |||
| − | |||
| − | + | ===비에타의 공식=== | |
| − | + | * 1593년 François Viète에 의해 발견된 [[비에타의 공식]] | |
| + | * 원주율의 무한곱 표현:<math>\frac{2}{\pi}=\frac{\sqrt{2}}{2}\frac{\sqrt{2+\sqrt{2}}}{2} \frac{\sqrt{2+\sqrt{2+\sqrt{2}}}}{2} \frac{\sqrt{2+\sqrt{2+\sqrt{2+\sqrt{2}}}}}{2} \frac{\sqrt{2+\sqrt{2+\sqrt{2+\sqrt{2+\sqrt{2}}}}}}{2} \frac{\sqrt{2+\sqrt{2+\sqrt{2+\sqrt{2+\sqrt{2+\sqrt{2}}}}}}}{2}\cdots</math> | ||
| − | + | ||
| − | + | ||
| − | + | ===급수표현=== | |
| − | + | * 1680년경에 발견된 [[그레고리-라이프니츠 급수|라이프니츠 급수]] | |
| + | :<math>1 \,-\, \frac{1}{3} \,+\, \frac{1}{5} \,-\, \frac{1}{7} \,+\, \frac{1}{9} \,-\, \cdots \;=\; \frac{\pi}{4}</math> | ||
| − | + | ||
| − | + | ||
| − | + | ===마친의 공식=== | |
| − | + | * 1706년 발견된 [[마친(Machin)의 공식]]:<math>\frac{\pi}{4}=4\alpha-\beta=4\arctan \frac{1}{5}-\arctan \frac{1}{239}</math> | |
| − | + | ||
| + | |||
| − | + | ===산술기하평균함수와 파이=== | |
| − | |||
| − | |||
| − | + | * [[산술기하평균함수(AGM)와 파이값의 계산|산술기하평균함수(AGM)와 파이)]] | |
| − | + | ||
| − | + | ||
| − | + | ===라마누잔의 공식=== | |
| − | + | * 라마누잔은 1914년에 다음과 같은 공식을 발표:<math>\frac{1}{\pi}= \frac{2\sqrt2}{9801}\sum_{n=0}^{\infty}\frac{(4n)!(1103+26390n)}{(n!)^{4}396^{4n}}</math> | |
| + | * 윌리엄 고스퍼는 1985년에 파이값의 천7백만자리를 계산하기 위해 이 공식을 사용 | ||
| + | * 비슷한 형태로 다음과 같은 공식:<math>\frac{426880 \sqrt{10005}}{\pi} = \sum_{k=0}^\infty \frac{(6k)! (13591409 + 545140134k)}{(3k)!(k!)^3 (-640320)^{3k}}\!</math> | ||
| + | * [[라마누잔과 파이]] 항목을 참조 | ||
| − | + | ==오일러와 파이== | |
| − | + | * [[오일러의 공식]]:<math>e^{i \pi} +1 = 0</math> | |
| + | * [[Ζ(2)의 계산, 오일러와 바젤문제(완전제곱수의 역수들의 합)]]:<math>\zeta(2)=\sum_{n=1}^{\infty}\frac{1}{n^2}=\frac{\pi^2}{6}=\frac{(2\pi)^2}{24}</math> | ||
| + | * 더 일반적으로 [[정수에서의 리만제타함수의 값]]은 다음과 같이 주어진다:<math>\zeta(2n) =(-1)^{n+1}\frac{B_{2n}(2\pi)^{2n}}{2(2n)!}, n \ge 1</math>여기서 <math>B_{2n}</math>은 [[베르누이 수|베르누이수]]. | ||
| − | + | ||
| + | |||
| − | + | ==BBP 공식== | |
| + | * [[원주율의 BBP 공식|BBP 공식]] 항목 참조 | ||
| + | :<math>\pi = \sum_{k = 0}^{\infty}\frac{1}{16^k} \left( \frac{4}{8k + 1} - \frac{2}{8k + 4} - \frac{1}{8k + 5} - \frac{1}{8k + 6} \right)</math> | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | ==complex multiplication과 파이== | |
| − | === | + | * 타원곡선의 [[complex multiplication]] 이론과 [[타원 모듈라 j-함수 (elliptic modular function, j-invariant)|타원 모듈라 j-함수 (j-invariant)]]는 다음과 같은 공식들을 이해할 수 있게 해준다:<math>\large e^{\pi \sqrt{163}}=262537412640768743.9999999999992500725\cdots\approx 262537412640768744</math>:<math>e^{\pi \sqrt{43}} = 884736743.9997774660349066619374620785\approx 884736744</math>:<math>e^{\pi \sqrt{67}} = 147197952743.9999986624542245068292613\approx 147197952744</math> |
| + | * [[숫자 163]], [[숫자 67]] 항목과 [[가우스의 class number one 문제]] | ||
| − | + | ||
| − | |||
| − | + | ||
| − | + | ==파이가 아니라 2파이다?== | |
| − | < | + | * 수학의 많은 공식에서는 <math>\pi</math>가 아닌 <math>2\pi</math>가 자연스럽게 등장 |
| + | * [[파이가 아니라 2파이다?]] | ||
| − | + | ||
| − | + | ||
| − | + | ==메모== | |
| − | + | * http://navercast.naver.com/science/math/1094 | |
| + | * http://navercast.naver.com/science/math/204 | ||
| − | + | ==관련된 항목들== | |
| − | + | * [[너드의 길]] | |
| − | + | * [[오일러의 공식]] | |
| − | * | + | * [[ζ(2)의 계산, 오일러와 바젤문제(완전제곱수의 역수들의 합)]] |
| − | + | * [[정수에서의 리만제타함수의 값]] | |
| − | + | * [[라마누잔과 파이]] | |
| − | + | * [[파이(영화)]] | |
| − | + | * [[삼각함수와 쌍곡함수의 맥클로린 급수]] | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | * [ | ||
| − | * | ||
| − | * | ||
| − | |||
| − | * | ||
| − | * | ||
| − | * | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | ||
| − | + | ==계산 리소스== | |
| + | * http://oeis.org/A000796 | ||
| + | * http://www.wolframalpha.com/input/?i=pi | ||
| + | * [http://math.fullerton.edu/mathews/n2003/picomputation/PiComputationBib/Links/PiComputationBib_lnk_ 2.html Bibliography for Computation of Pi] | ||
| − | + | ||
| − | + | ==사전 형태의 자료== | |
| − | * http:// | + | * http://ko.wikipedia.org/wiki/원주율 |
| − | + | * http://en.wikipedia.org/wiki/ | |
| − | * http:// | ||
| − | |||
| − | |||
| − | + | ||
| − | + | ||
| − | + | ==관련논문== | |
| + | * Bailey, David H., Simon M. Plouffe, Peter B. Borwein, and Jonathan M. Borwein. “The Quest for PI.” The Mathematical Intelligencer 19, no. 1 (December 1, 1997): 50–56. doi:10.1007/BF03024340. http://crd.lbl.gov/%7Edhbailey/dhbpapers/pi-quest.pdf | ||
| + | * Borwein, Jonathan M. “The Life of Π: From Archimedes to ENIAC and Beyond.” In From Alexandria, Through Baghdad, edited by Nathan Sidoli and Glen Van Brummelen, 531–61. Springer Berlin Heidelberg, 2014. http://link.springer.com/chapter/10.1007/978-3-642-36736-6_24. | ||
| + | * Schepler, Herman C. “The Chronology of PI.” Mathematics Magazine 23, no. 4 (March 1, 1950): 216–28. doi:10.2307/3029832. http://www.jstor.org/stable/3029832 | ||
| − | + | ==관련도서== | |
| − | |||
| − | + | * [http://www.aladdin.co.kr/shop/wproduct.aspx?isbn=8981727937 수학도깨비에게 원주율 배우기] | |
| + | ** 최행진, 교우사, 2009-03-05 | ||
| + | * [http://www.yes24.com/24/goods/340156 파이의 즐거음] | ||
| + | ** 데이비드 블래트너, 2003 | ||
| + | * [http://books.google.com/books?id=QwwcmweJCDQC Pi-unleashed] | ||
| + | ** Jörg Arndt, Christoph Haenel, C. Lischka, D. Lischka, 2000 | ||
| + | * [http://www.amazon.com/PI-AGM-Analytic-Computational-Complexity/dp/047131515X Pi and the AGM] | ||
| + | ** Jonathan M. Borwein, Peter B. Borwein, 1998 | ||
| − | + | ||
| − | + | ||
| − | |||
| − | |||
| − | + | ==관련기사== | |
| − | + | * 네이버 뉴스 검색 (키워드 수정) | |
| + | ** http://news.search.naver.com/search.naver?where=news&x=0&y=0&sm=tab_hty&query=원주율 | ||
| + | ** http://news.search.naver.com/search.naver?where=news&x=0&y=0&sm=tab_hty&query=파이데이 | ||
| + | [[분류:원주율]] | ||
| + | [[분류:상수]] | ||
| − | * | + | ==메타데이터== |
| + | ===위키데이터=== | ||
| + | * ID : [https://www.wikidata.org/wiki/Q4897101 Q4897101] | ||
| + | ===Spacy 패턴 목록=== | ||
| + | * [{'LOWER': 'chronology'}, {'LOWER': 'of'}, {'LOWER': 'computation'}, {'LOWER': 'of'}, {'LEMMA': 'π'}] | ||
2021년 2월 17일 (수) 05:55 기준 최신판
개요
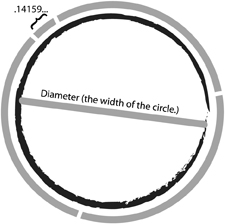
- 원주율(파이,π)는 원의 둘레와 지름의 비율
- 모든 원은 서로 닮음이므로, 이 비율은 상수이다
- \(\pi=3.141592653589793238462643383279502884197169399375\cdots\)
- 수학의 수많은 곳에서 등장한다
표기법의 역사
- http://collation.folger.edu/2015/03/pi-day-represented/
- The first time we see the Greek letter π used in connection with circles is in Oughtred’s 1647 Key of the Mathematics. Here, Oughtred used π to represent the periphery (or circumference) of a circle and ∂ to represent the diameter in the ratio.
- It wasn’t until the beginning of the 18th century that π started to be used in its current way, thanks to to William Jones’s A New Introduction to the Mathematics
원주율의 계산
- http://numbers.computation.free.fr/Constants/Pi/piCompute.html
- http://en.wikipedia.org/wiki/Chronology_of_computation_of_%CF%80
아르키메데스의 부등식
- \(223/71 < \pi < 22/7\)
비에타의 공식
- 1593년 François Viète에 의해 발견된 비에타의 공식
- 원주율의 무한곱 표현\[\frac{2}{\pi}=\frac{\sqrt{2}}{2}\frac{\sqrt{2+\sqrt{2}}}{2} \frac{\sqrt{2+\sqrt{2+\sqrt{2}}}}{2} \frac{\sqrt{2+\sqrt{2+\sqrt{2+\sqrt{2}}}}}{2} \frac{\sqrt{2+\sqrt{2+\sqrt{2+\sqrt{2+\sqrt{2}}}}}}{2} \frac{\sqrt{2+\sqrt{2+\sqrt{2+\sqrt{2+\sqrt{2+\sqrt{2}}}}}}}{2}\cdots\]
급수표현
- 1680년경에 발견된 라이프니츠 급수
\[1 \,-\, \frac{1}{3} \,+\, \frac{1}{5} \,-\, \frac{1}{7} \,+\, \frac{1}{9} \,-\, \cdots \;=\; \frac{\pi}{4}\]
마친의 공식
- 1706년 발견된 마친(Machin)의 공식\[\frac{\pi}{4}=4\alpha-\beta=4\arctan \frac{1}{5}-\arctan \frac{1}{239}\]
산술기하평균함수와 파이
라마누잔의 공식
- 라마누잔은 1914년에 다음과 같은 공식을 발표\[\frac{1}{\pi}= \frac{2\sqrt2}{9801}\sum_{n=0}^{\infty}\frac{(4n)!(1103+26390n)}{(n!)^{4}396^{4n}}\]
- 윌리엄 고스퍼는 1985년에 파이값의 천7백만자리를 계산하기 위해 이 공식을 사용
- 비슷한 형태로 다음과 같은 공식\[\frac{426880 \sqrt{10005}}{\pi} = \sum_{k=0}^\infty \frac{(6k)! (13591409 + 545140134k)}{(3k)!(k!)^3 (-640320)^{3k}}\!\]
- 라마누잔과 파이 항목을 참조
오일러와 파이
- 오일러의 공식\[e^{i \pi} +1 = 0\]
- Ζ(2)의 계산, 오일러와 바젤문제(완전제곱수의 역수들의 합)\[\zeta(2)=\sum_{n=1}^{\infty}\frac{1}{n^2}=\frac{\pi^2}{6}=\frac{(2\pi)^2}{24}\]
- 더 일반적으로 정수에서의 리만제타함수의 값은 다음과 같이 주어진다\[\zeta(2n) =(-1)^{n+1}\frac{B_{2n}(2\pi)^{2n}}{2(2n)!}, n \ge 1\]여기서 \(B_{2n}\)은 베르누이수.
BBP 공식
- BBP 공식 항목 참조
\[\pi = \sum_{k = 0}^{\infty}\frac{1}{16^k} \left( \frac{4}{8k + 1} - \frac{2}{8k + 4} - \frac{1}{8k + 5} - \frac{1}{8k + 6} \right)\]
complex multiplication과 파이
- 타원곡선의 complex multiplication 이론과 타원 모듈라 j-함수 (j-invariant)는 다음과 같은 공식들을 이해할 수 있게 해준다\[\large e^{\pi \sqrt{163}}=262537412640768743.9999999999992500725\cdots\approx 262537412640768744\]\[e^{\pi \sqrt{43}} = 884736743.9997774660349066619374620785\approx 884736744\]\[e^{\pi \sqrt{67}} = 147197952743.9999986624542245068292613\approx 147197952744\]
- 숫자 163, 숫자 67 항목과 가우스의 class number one 문제
파이가 아니라 2파이다?
- 수학의 많은 공식에서는 \(\pi\)가 아닌 \(2\pi\)가 자연스럽게 등장
- 파이가 아니라 2파이다?
메모
관련된 항목들
- 너드의 길
- 오일러의 공식
- ζ(2)의 계산, 오일러와 바젤문제(완전제곱수의 역수들의 합)
- 정수에서의 리만제타함수의 값
- 라마누잔과 파이
- 파이(영화)
- 삼각함수와 쌍곡함수의 맥클로린 급수
계산 리소스
- http://oeis.org/A000796
- http://www.wolframalpha.com/input/?i=pi
- 2.html Bibliography for Computation of Pi
사전 형태의 자료
관련논문
- Bailey, David H., Simon M. Plouffe, Peter B. Borwein, and Jonathan M. Borwein. “The Quest for PI.” The Mathematical Intelligencer 19, no. 1 (December 1, 1997): 50–56. doi:10.1007/BF03024340. http://crd.lbl.gov/%7Edhbailey/dhbpapers/pi-quest.pdf
- Borwein, Jonathan M. “The Life of Π: From Archimedes to ENIAC and Beyond.” In From Alexandria, Through Baghdad, edited by Nathan Sidoli and Glen Van Brummelen, 531–61. Springer Berlin Heidelberg, 2014. http://link.springer.com/chapter/10.1007/978-3-642-36736-6_24.
- Schepler, Herman C. “The Chronology of PI.” Mathematics Magazine 23, no. 4 (March 1, 1950): 216–28. doi:10.2307/3029832. http://www.jstor.org/stable/3029832
관련도서
- 수학도깨비에게 원주율 배우기
- 최행진, 교우사, 2009-03-05
- 파이의 즐거음
- 데이비드 블래트너, 2003
- Pi-unleashed
- Jörg Arndt, Christoph Haenel, C. Lischka, D. Lischka, 2000
- Pi and the AGM
- Jonathan M. Borwein, Peter B. Borwein, 1998
관련기사
- 네이버 뉴스 검색 (키워드 수정)
메타데이터
위키데이터
- ID : Q4897101
Spacy 패턴 목록
- [{'LOWER': 'chronology'}, {'LOWER': 'of'}, {'LOWER': 'computation'}, {'LOWER': 'of'}, {'LEMMA': 'π'}]