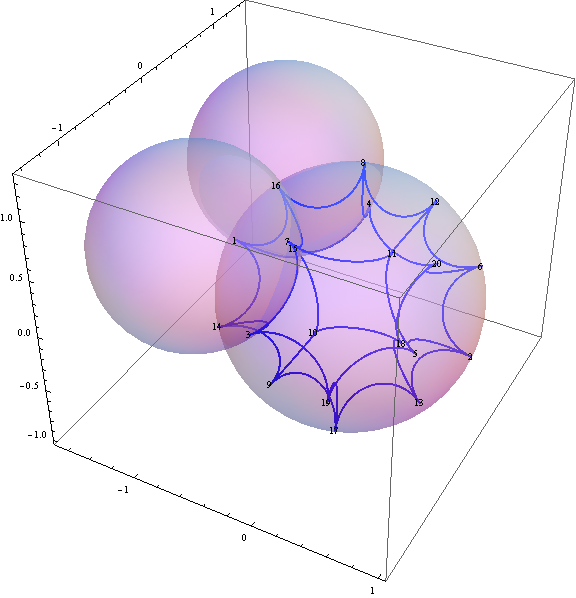
쌍곡 정십이면체

개요

• 3차원 푸앵카레 unit ball 모델에서의 쌍곡 정십이면체
• 꼭지점들이 unit ball에 놓여 있는 경우 (ideal hyperbolic regular dodecahedron)

쌍곡 다양체로서의 부피

• \(20.5801993539\cdots\)

메모

• http://www.bugman123.com/Hyperbolic/
• http://mathematica.stackexchange.com/questions/20225/how-do-i-generate-the-mathematica-version-2-spikey-in-mathematica
• The hyperbolic chamber

관련된 항목들

• 쌍곡 정육면체
• 정다면체
• 로바체프스키 함수
• 푸앵카레 정십이면체 공간(dodecahedral space)

매스매티카 파일 및 계산 리소스

• https://docs.google.com/file/d/0B8XXo8Tve1cxelRkc3RDVGs2RmM/edit
• Michael Trott, Making the Mathematica 6 Spikey, 2007
  • The Cover Image: Making the Mathematica 6 Surface-Textured Hyperbolic Dodecahedron
• http://mathworld.wolfram.com/HyperbolicDodecahedron.html

에세이

• Vladimir Bulatov, Tilings of the hyperbolic space and their visualization

관련논문

• Szirmai, Jenő. 2013. “The Optimal Ball and Horoball Packings of the Coxeter Tilings in the Hyperbolic 3-space.” Beiträge Zur Algebra Und Geometrie 46 (2): 545–558.
• Kozma, Robert Thijs, and Jenő Szirmai. 2012. “Optimally Dense Packings for Fully Asymptotic Coxeter Tilings by Horoballs of Different Types.” Monatshefte Für Mathematik 168 (1) (October 1): 27–47. doi:10.1007/s00605-012-0393-x.